When there is no prior knowledge on the fatigue case, a suitable fatigue model can be proposed based on a few questions regarding loading conditions and expected fatigue failure. Figure 3-5 summarizes the key questions you should ask when evaluating fatigue using the Fatigue Module.

The stress history for random loads introduces a complex load scenario in the structure that requires an advanced evaluation technique to quantify the stress response. If your application is subjected to random loading, you can evaluate fatigue using the Cumulative Damage Model, where the random load is converted into a stress range distribution, rather than the single constant stress cycle — which is assumed for the other evaluation techniques.

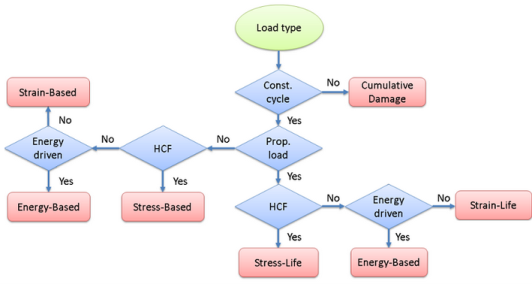
In the Fatigue Module, the Stress-Life Models and Strain-Life Models models can evaluate fatigue at proportional loading. These models are based on a fatigue-life curve, which provides a direct relation between the fatigue life and the applied stress or strain amplitude.

One model in the Stress-Life family requires extra attention: Approximate S-N Curve Model. In the model, you specify two points on the S-N curve. The first one is the transition between the high- and low-cycle fatigue, while the second defines the endurance limit. The advantage of this model is that it does not require any substantial knowledge of the fatigue material data, since the two required points can be related to the ultimate tensile strength. Although it is a rough approximation, it is a good starting point when you lack material data.

In the Fatigue Module, this type of application can be assessed with the Strain-Based Fatigue Models and Stress-Based Fatigue Models. These are Critical Plane Methods because they evaluate many orientations in space in search for the critical plane where fatigue is expected to occur.

The Strain-Based Fatigue Models are suitable for fatigue prediction at low-cycle fatigue, while the Stress-Based Fatigue Models are frequently used to predict high-cycle fatigue. Most of the fatigue models predict the number of cycles until failure. The Stress-Based Fatigue Models predict a fatigue usage factor, which is the fraction between the applied stress and the stress limit. This indicates to the user whether the stress limit has been exceeded and failure is expected or if the component will hold for the expected fatigue life. You can view the fatigue usage factor as the inverse of a safety factor.

Several of the Stress-Based and the Strain-Based models incorporate normal stress fatigue sensitivity. The Dang Van model from the Stress-Based family takes into account the compressive stress instead and is therefore suitable for contact fatigue analysis.

In some cases, the stress or strain alone is not sufficient to characterize the fatigue properties. You can then use the Energy-Based Fatigue Models. These combine the effect of stress and strain into energy, which is released or dissipated during a load cycle.

The Energy-Based Fatigue Models are frequently used in nonlinear materials in the low-cycle fatigue regime. Since the energy can be calculated in different ways, the Energy-Based Fatigue Models can be used in both proportionally and non-proportionally loaded applications.