
|
|
1
|
|
2
|
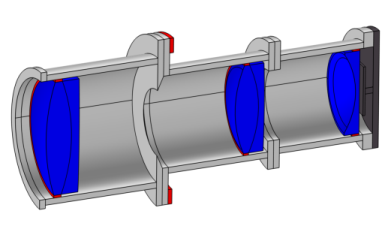
In the Application Libraries window, select Ray Optics Module>Structural Thermal Optical Performance Analysis>petzval_lens_stop_analysis in the tree.
|
|
3
|
Click
|
|
1
|
|
1
|
|
2
|
|
3
|
|
4
|
|
5
|
Locate the Hyperelastic Material section. From the Compressibility list, choose Incompressible material.
|
|
6
|
|
1
|
|
2
|
|
1
|
|
2
|
|
3
|
|
4
|
|
5
|
|
6
|
|
7
|