The virtual mass force is an optional term that can be defined by the Drag Force node by clicking the Include virtual mass and pressure gradient forces check box.

For a particle of velocity v (SI unit: m/s) in a fluid of velocity u (SI unit: m/s), the virtual mass term Fvm (SI unit: N) is (Ref. 19)

where mf (SI unit: kg) is the mass of the fluid displaced by the particle volume,

dp (SI unit: m) is the particle diameter, and ρ (SI unit: kg/m3) is the density of the fluid at the particle’s position.

The derivative term in Equation 5-12 is a total derivative or material derivative. Therefore it considers not only the time dependence of the velocity at a fixed point, but also the motion of the particle relative to the fluid. For an arbitrary scalar field f, the material derivative is defined as

Alternatively, for an arbitrary vector field f, the material derivative is

where becomes a rank 2 tensor. In either case, the dot product is taken with the particle velocity v instead of the fluid velocity u, following Ref. 19.

Using the above definition of the material derivative, Equation 5-12 becomes

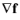
Since v is the velocity of a discrete particle, not a field variable, its gradient vanishes, leaving only

The term involving the time derivative of particle velocity v can be moved to the left-hand side of the equation of motion, by manipulating the equation of motion of a particle as follows:

where mv (SI unit: kg) is the virtual mass,

To summarize, when including the virtual mass force, the particle mass mp on the left-hand side of the equation of motion is replaced with the virtual mass mv. The contribution of the virtual mass force to the right-hand side then contains only the material derivative of the fluid velocity u,

If the virtual mass force is included in a model and the components of the virtual mass force are evaluated during results processing (for example a Particle Evaluation with the expression fpt.df1.Fvmx), the expression being evaluated will be Equation 5-13, not Equation 5-12.