The term shape memory alloy (SMA) normally refers to alloys that can undergo large strains, effect called
superelasticity or
pseudoelasticity; and also to materials that after undergoing large deformations can remember their original shape when heated above a certain temperature.
Cold NiTi alloys are composed by 100% of martensite volume fraction, ξM. The austenite phase develops upon heating the material above the
austenite start temperature,
As. If the alloy is heated above the
austenite finish temperature,
Af, the alloy microstructure becomes 100% of austenite. The reverse process has different transition temperatures: If a 100% austenite alloy is cooled below the
martensite start temperature,
Ms, the martensite volume fraction
ξM will develop until the cooling temperature reaches the
martensite finish temperature,
Mf, below which the alloy microstructure will be pure martensite.
here, c is the heat capacity at constant pressure,
K and
G are the bulk and shear moduli,
εvol is the volumetric strain,
Hk is the hardening modulus, and
I(
εtr) is the
indicator function for the strain limit constraint. The
effective transformation strain εtre is used as a measure of the transformation strain tensor
The term ψch = f(T)|εtr| is denoted as the chemical energy density due to the thermally induced martensite transformation. The function
f(
T) corresponds to the temperature-dependent martensite to austenite equilibrium stress, define from the slope of the limit curve
β and the martensite finish temperature
Mf
Here, the operator < . > denotes the Macaulay brackets.
The conjugated thermodynamic stress σtr associated to the transformation strain variable is
The evolution of the transformation strain εtr is given by the so-called
limit function, which takes the same form as the yield function for metal plasticity.
where the plastic multiplier λp is solved with the Kuhn-Tucker conditions, as done for plasticity, see
Plastic Flow for Small Strains.
For Lagoudas model, Gibbs free energy density depends on two state variables: the total stress tensor σ and the temperature field
T. Additional internal variables are used to compute the transformation strain tensor
εtr and the martensite volume fraction
ξ (
Ref. 20)
Here, c is the heat capacity at constant pressure,
s0 is the specific entropy at reference state,
S is the compliance matrix,
εth is the thermal strain tensor,
εtr is the transformation strain tensor,
u0 is the specific internal energy at reference state, and
f(
ξ) is the
transformation hardening function. The compliance matrix
S, is obtained by averaging the elastic properties of martensite and austenite
where ΔS = SM − SA. Also, other material parameters are averaged this way.
where the normalized transformation tensor Λ changes principal directions depending on the direction of the martensitic transformation.
The maximum transformation strain in Lagoudas model can be considered constant, or stress-dependent as described in Ref. 21. A stress-dependent maximum transformation strain can be used at low stress levels, where the martensite turn into detwinned structures.
The variable for the direction of the martensitic transformation,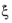 
, is calculated from the previous state to determine the expected increment or decrement of the transformation strain tensor εtr. This calculation is computational expensive and it can lead to convergence issues. Since in many applications the transformation direction
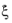
is known a priori (for instance, mechanical loading or unloading, or temperature increment/decrement) a user input enables to set the transformation direction manually to 1 or -1, thus speeding up the computational time.
and 
, 
and 
