Consider the molecules effused from an infinite half space filled with a gas of number density n through a small hole in the half space with diameter much less than the mean free path of the gas.
Within the gas the molecules are traveling in all directions on a unit sphere with equal probability. The probability of molecules striking the hole at angles between θ and
θ + dθ to the normal of the bounding surface of the half space is proportional to the fraction of solid angle (or angle in 2D) that this angular range occupies. In 3D, as shown in
Figure 3-3, the total solid angle corresponding to angles between
θ and
θ + dθ and
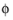
and
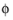 + d
+ d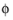
is
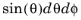
. In 2D the angle is simply
dθ.
If molecules are traveling toward the surface at velocity c,
then a volume of gas
Ac cos θ strikes area
A in unit time. The effusing flux contribution is then
nc cos θ. The contribution to the total flux from molecules at angles between
θ and
θ + dθ with speeds
c and
c + dc is therefore: