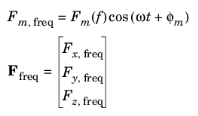Frequency-Domain Studies
In the frequency domain, the frequency response is studied when applying harmonic loads. Harmonic loads are specified using two components:
•
The amplitude value in direction m, Fm
•
The corresponding phase, ϕm
To derive the equations for the linear response from harmonic excitation loads
Assume a harmonic response with the same angular frequency as the excitation load
The relationship can also be described using complex notation with
and
The primary results, such as displacements, velocities, accelerations, and stress and strain components are all complex valued.
Cycle Maximum
Quantities that are nonlinear with respect to the degrees of freedom will in general not have a harmonic variation. In some cases, it is however possible to derive maximum values for a cycle. The norm of a vector is defined as
The components of the vector can have different phases, so that the temporal variation is
where aj is the amplitude, and φj is the phase angle. Then,
The square of the norm is thus a harmonic function with double the excitation frequency. The harmonic term can, using standard trigonometric expressions be rewritten as
where
The maximum value of the vector norm during the cycle is thus
The expressions above can be generalized to any quadratic form of the type
In particular, the von Mises equivalent stress is of this form, with
Using the same development as above,
This expression can be split into a constant (period average) term, and a periodic term, so that
where
and
The maximum value of the norm is thus
Since, for the von Mises stress, the matrix c is symmetric and have many zero elements, the number of terms in the sums that actually contribute are far less than the nominal number.
When computing the cycle maximum of the von Mises stress, there is an alternative, simpler approach. The von Mises stress can be written as
where s is the deviatoric stress defined as
Note that on this form, there are no cross products between different components of the tensor. By defining
the expression for the maximum of a vector norm can be used directly to compute the maximum von Mises stress.