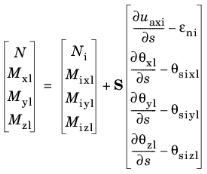Theory for Section Stiffness

The Section Stiffness node in the Beam interface allows modeling of beams with nonhomogeneous cross sections by, instead of geometrical and material properties, directly entering values for the stiffness matrix S.

For a 3D Euler-Bernoulli beam, the relationship between the section forces and the deformation of the beam is given by

(8-1)

including initial normal force Ni; moments Mixl, Miyl, and Mizl; normal strain εi; and curvatures θsixl, θsiyl, and θsizl. Here, S is a 4-by-4 symmetric matrix. For a homogeneous prismatic beam with isotropic material properties, it is defined as

For a 2D Euler-Bernoulli beam, out-of-plane moments are zero, and Equation 8-1 thus simplifies to

with S now being a 2-by-2 symmetric matrix.

For a 3D Timoshenko beam, the relationship between the section forces and the deformation of the beam is given by

(8-2)

with S being a 6-by-6 symmetric matrix. The equation now also includes the initial shear forces Tiyl and Tizl as well as the initial shear strains γiyl and γizl.

For a 2D Timoshenko beam, out-of-plane forces and moments are zero, and Equation 8-2 thus simplifies to

with S now being a 3-by-3 symmetric matrix.

Using the section forces, the virtual work for the beam becomes

where only the relevant terms are kept, depending on the beam formulation and the space dimension.