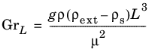In cases of externally driven flow, such as forced convection, the nature of the flow is characterized by the Reynolds number, Re, which describes the ratio of the inertial forces to the viscous forces. However, the velocity scale is initially unknown for internally driven flows such as natural convection. In such cases the Grashof number,
Gr, characterizes the flow. It describes the ratio of the time scales for viscous diffusion in the fluid and the internal driving force (the buoyancy force). Like the Reynolds number it requires the definition of a length scale, the fluid’s physical properties, and the density scale (densities difference).
where g is the acceleration of gravity,
ρs denotes the density of the hot surface,
ρext equals the free stream density,
L is the length scale,
μ represents the fluid’s dynamic viscosity, and
ρ its density.
where g is the acceleration of gravity,
Ts denotes the temperature of the hot surface,
Text equals the free stream temperature,
L is the length scale,
μ represents the fluid’s dynamic viscosity, and
ρ its density.
The transition from laminar to turbulent flow occurs at a Gr value of
109; the flow is turbulent for larger values.
The Rayleigh number, Ra, is another indicator of the regime. It is similar to the Grashof number except that it accounts for the thermal diffusivity:
Ra = Pr Gr. A small value of the
Ra number indicates that the conduction dominates. It such case using heat transfer coefficients to model convective heat transfer is not relevant. Instead, modeling the fluid as immobile is likely to be accurate.