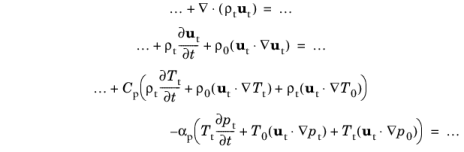The Nonlinear Thermoviscous Acoustics Contributions feature adds the necessary contributions to the governing
Equation 6-1 in order to model nonlinear effects in a transient thermoviscous simulation. The contributions allow modeling of vortex shedding that may happen at sudden expansions, like in a perforate, a grill, or at a miniature sound port. Vortex shedding will in general introduce distortion in the measured response of a system, with the generation of harmonics. The feature can also capture the nonlinear effect associated with high sound pressure levels that require a nonlinear representation of the equation of state (pressure, density, and temperature relation). The nonlinear contributions to the left-hand side of
Equation 6-1 are:
For systems where the linearity condition on the density ρt <<
ρ0 is no longer fulfilled, the density expansion can be changed to include second order terms (see below). This will allow modeling of so-called cumulative effects.
The Nonlinear Thermoviscous Acoustics Contributions feature is not compatible with the
Background Acoustic Fields feature. The superposition principle is not valid in a nonlinear model.
Note that when solving nonlinear models, it is often necessary to use numerical stabilization. Turn it on in the Stabilization section. Per default no stabilization is used. Using stabilization also allows to switch to a P1-P1-P1 discretization which can be more efficient in transient models. Remember to use an adequate mesh for a lower order discretization, especially in the acoustic boundary layers.
The model inputs for the Equilibrium pressure p0 and the
Equilibrium temperature T0 are always visible as they contribute to the governing equations.
When Second order is selected, additional inputs to the model are necessary. For the general case, the second order derivatives of the equilibrium density
ρ0 =
ρ0(
p0,
T0) with respect to pressure
p0 an temperature are necessary
T0 are needed. They contribute to the second-order Taylor expansion of the density. Per default, they are taken
From equilibrium density; this implies that the dependency of the density on pressure and temperature should be correct.
If the Adiabatic formulation is used (see
Thermoviscous Acoustics Equation Settings), the user interface inputs correspond to the
Nonlinear Acoustics (Westervelt) Contributions equation in Pressure Acoustics, Transient. Select to specify the
Parameter of nonlinearity (default), the
Ratio of specific heats (for gases), or the
Coefficient of nonlinearity.
Click to select Include viscous dissipation (disabled per default). This will add a right-hand side heat source to the energy equation. The viscous dissipation is a nonlinear (second order) effect and can only be included in the nonlinear model.