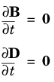COMSOL 6.2 - Forces in Stationary Fields

Also assume that the objects modeled are not moving v = 0 so that there is no contributions from Lorentz forces. These are treated later on.

	To apply the stress tensor in air to calculate the total force and torque on a magnetizable rod close to a permanent magnet, see Permanent Magnet: Application Library path ACDC_Module/Introductory_Magnetostatics/permanent_magnet.

The expressions for the stress tensor in a general electromagnetic context stems from a fusion of material theory, thermodynamics, continuum mechanics, and electromagnetic field theory. With the introduction of thermodynamic potentials for mechanical, thermal, and electromagnetic effects, explicit expressions for the stress tensor can be derived in a convenient way by forming the formal derivatives with respect to the different physical fields (Ref. 1 and Ref. 3). Alternative derivations can be made for a vacuum (Ref. 4) but these cannot easily be generalized to polarized and magnetized materials.

For air, the stress tensor is

where p is the air pressure, I is the identity 3-by-3 tensor (or matrix), and E and B are 3-by-1 vectors. In this expression of the stress tensor, air is considered to be nonpolarizable and nonmagnetizable. When air is approximated by vacuum, p = 0. This expression, with p = 0, of the stress tensor is also known as the Maxwell stress tensor.

Using the fact that, for air, D = ε0E and B = μ0H the expression for the stress tensor can be written as

The equation for the balance of forces becomes

Maxwell’s equations in free space give that the contribution of the electromagnetic part of the stress tensor is zero, and the resulting expression is

Thus, using the same terminology as earlier, fem = 0 for air, with σM = −pI. In the derivation of the total force on an elastic solid surrounded by vacuum or air, the approximation ∇p = 0 has been used.

When operating with the divergence operator on the stress tensor, the relation

is useful (and similarly for B). From the right-hand side it is clear (using Maxwell’s equations) that this is zero for stationary fields in free space.

Consider again the case of a solid surrounded by air. To compute the total force, the projection of the stress tensor on the outside of the solid surface is needed,

where n1 is the surface normal, a 1-by-3 vector, pointing out from the solid. This expression can be used directly in the boundary integral of the stress tensor for calculating the total force F on the solid.

	For an example of how to compute the total force on two parallel wires either by integrating the volume force or by integrating the stress tensor on the surrounding surface, see Electromagnetic Forces on Parallel Current-Carrying Wires: Application Library path ACDC_Module/Introductory_Electromagnetic_Forces/parallel_wires.

A material that is nonpolarizable and nonmagnetizable (P = 0 and M = 0) is called a pure conductor. This is not necessarily equivalent to a perfect conductor, for which E = 0, but merely a restriction on the dielectric and magnetic properties of the material. The stress tensor becomes identical to the one for air, except for −pI being replaced by the purely mechanical stress tensor σM:

where D = ε0E and B = μ0H.

The situation is slightly different from the case of air because there can be currents and volume charges in the conductor. The current density is

and the volume charge density

The equation for the balance of forces now becomes

For an elastic solid, in the general case of a material that is both dielectric and magnetic (nonzero P and M), the stress tensor is given by the expression

where in σ(E, B) the dependence of E and B has not been separated out. Thus σ is not a purely mechanical stress tensor in this general case. Different material models give different appearances of σ(E, B). The electromagnetic contributions to σ(E, B) typically represent pyroelectric, pyromagnetic, piezoelectric, piezomagnetic, dielectric, and magnetization effects. The expression for the stress tensor in vacuum, air, and pure conductors can be derived from this general expression by setting M = P = 0.

T1 must be symmetric. The terms EPT and −MBT are symmetric in the case of a linear dielectric and magnetic material because

Here, the magnetic susceptibility χB differs slightly from the classical χm. The other explicit terms are all symmetric, as is σ(E, B). In the general case this imposes constraints on the properties of σ(E, B). For a nonlinear material σ(E, B) might need to include terms such as −EPT or +MBT to compensate for asymmetric EPT or −MBT.