In the Soil Plasticity subnode you define the properties for modeling materials exhibiting soil plasticity. Soil Plasticity can be used together with Linear Elastic Material and Nonlinear Elastic Material. It is available with the Geomechanics Module. Soil Plasticity is available for 3D, 2D, and 2D axisymmetry.

Select the Material model — Drucker-Prager, Mohr-Coulomb, Matsuoka-Nakai, or Lade-Duncan. Most values are taken From material. For User defined choices, enter other values or expressions.

In the standard Drucker–Prager formulation, the material parameters are given in terms of the α and k coefficients. Often material data is expressed in the parameters c and φ used in the Mohr–Coulomb model. You can then choose to use there parameters instead. If so, select the Match to Mohr-Coulomb criterion check box (see The Mohr–Coulomb Criterion). If this check box is selected, the default values for Cohesion c and the Angle of internal friction 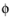are taken From material.

If required, select the Use dilatation angle in plastic potential check box. If this check box is selected, then enter a value or expression for the Dilatation angle ψ. Alternatively, select From material. The dilatation angle replaces the angle of internal friction when defining the plastic potential.

If the Match to Mohr-Coulomb criterion check box is not selected, then the default Drucker-Prager alpha coefficient and Drucker-Prager k coefficient are taken From material.

If required, select the Include elliptic cap check box. Select from the list the hardening model. When Perfectly plastic (no hardening) is selected, enter values or expressions to define the semi-axes of the ellipse under Elliptic cap parameter pa and Elliptic cap parameter pb. When Isotropic hardening is selected from the list, the default Isotropic hardening modulus Kiso, the Maximum plastic volumetric strain εpvol,max, and the Ellipse aspect ratio R are taken From material (see Elliptic Cap With Hardening). Enter a value or expression to define the initial semi-axis of the ellipse under the Initial location of the cap pb0.

The default Angle of internal friction 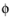and Cohesion c are taken From material.

If required, select the Use dilatation angle in plastic potential check box. If this check box is selected, then enter a value or expression for the Dilatation angle ψ. Alternatively, select From material. The dilatation angle replaces the angle of internal friction when defining the plastic potential.

Under Plastic potential select either Drucker-Prager matched at compressive meridian, Drucker-Prager matched at tensile meridian, or Associated.

If required, select the Include elliptic cap check box. Select from the list the hardening model. When Perfectly plastic (no hardening) is selected, enter values or expressions to define the semi-axes of the ellipse under Elliptic cap parameter pa and Elliptic cap parameter pb. When Isotropic hardening is selected from the list, the default Isotropic hardening modulus Kiso, the Maximum plastic volumetric strain εpvol,max, and the Ellipse aspect ratio R are taken From material (see Elliptic Cap With Hardening). Enter a value or expression to define the initial semi-axis of the ellipse under the Initial location of the cap pb0.

If required, select the Match to Mohr-Coulomb criterion check box. If this check box is selected, the default Angle of internal friction 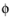is taken From material.

If the Match to Mohr-Coulomb criterion check box is not selected, then the default Matsuoka-Nakai mu coefficient μ is taken From material.

If required, select the Match to Mohr-Coulomb criterion check box. If this check box is selected, then enter a value or expression for the Angle of internal friction 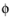. Alternatively, select From material.

If the Match to Mohr-Coulomb criterion check box is not selected, then the default Lade-Duncan k coefficient k is taken From material.

This section is only available with the Drucker-Prager and Mohr-Coulomb models

If required, select either None, Mean stress cutoff, or Principal stress cutoff.

When Mean stress cutoff is selected from the list, enter a value or expression for the Maximum mean stress σm. Use this to constrain the soil plasticity model with an extra yield surface which limits the maximum pressure in tension.

When Principal stress cutoff is selected from the list, enter a value or expression for the Maximum tensile stress σt. Use this to constrain the soil plasticity model with an extra yield surface, which limits the maximum principal tensile stress.

The default is None. Select Implicit Gradient to add nonlocal regularization to the equivalent plastic strain. Enter a value for the:

This section is available with the Implicit gradient nonlocal plasticity model. Select the shape function for the Nonlocal equivalent plastic strain εpe,nl — Automatic, Linear, Quadratic Lagrange, Quadratic serendipity, Cubic Lagrange, Cubic serendipity, Quartic Lagrange, Quartic serendipity, or Quintic Lagrange. The available options depend on the order of the displacement field.

To display this section, click the Show More Options button () and select Advanced Physics Options in the Show More Options dialog box.

To display this section, click the Show More Options button () and select Advanced Physics Options in the Show More Options dialog box.

Physics tab with Linear Elastic Material or Nonlinear Elastic Material node selected in the model tree: