Stability of a Turbocharger Under the Influence of Cross-Coupled Bearing Forces

A turbocharger is often supported by hydrodynamic journal bearings. Such bearings naturally have the cross-coupled forces present in them. These cross-coupled forces act as a negative damping in the system. Due to this, near the critical speed, the vibration amplitude in the turbocharger can become large, ultimately leading to the bearing failure. In this example we study the influence of these cross-coupled forces on the dynamics of the rotor.

Variation in the eigenfrequencies and logarithmic decrement with the rotational speed of the rotor gives the idea of the stability state of the overall system. Response of the turbocharger due to external forces at the turbine and compressor is also studied. The waterfall diagram clearly shows that the response amplitude is maximum in the resonance conditions.

Model Definition

The model consists of a turbocharger rotor supported by two bearings, one near the compressor and another near turbine, making both the compressor and turbine overhung on the shaft. The geometry of the rotor is shown in Figure 1.

Figure 1: Rotor geometry.

Two different analyses are performed:

•

An eigenfrequency analysis.

•

A frequency response analysis for different angular speeds of the rotor. This analysis looks at the frequency spectrum of the rotor and how it changes with the rpm.

Case 1 — Eigenfrequency Analysis

In this case, eigenfrequency analysis is performed for the different angular speeds of the turbocharger. Structural damping is added to damp the high frequency vibrations of the rotor. The bearings are modeled by equivalent stiffness and damping constants. Bending stiffness and damping in the bearings are neglected.

Properties of the bearings used in this analysis are given in Table 1:

Table 1: Bearing properties.
Property	Value
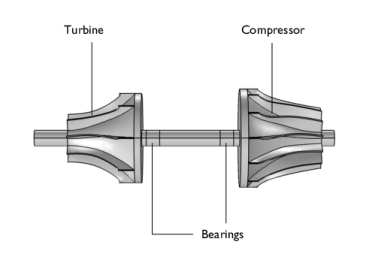
kyy (N/m)	1·108
kzz (N/m)	1·108
kyz (N/m)	4·107
kzy (N/m)	-4·107

Two cases of the bearing stiffness are considered. In the first, the cross-coupled stiffness kyz and kzy are ignored and in the second all four components of the stiffness are present. The angular speed of the rotor is varied from 0 rpm to 100,000 rpm in steps of 5,000 rpm. The variations in natural frequencies and logarithmic decrements with angular speed of the rotor are analyzed.

CAse2 — Frequency response Analysis

In this case, you analyze the harmonic response of the turbocharger rotor due to the mass eccentricities at both turbine and compressor. The angular speed of the shaft is varied from 2,000 rpm to 100,000 rpm in steps of 2,000 rpm. The frequency is varied from 100 to 3000 Hz in steps of 100 Hz. Variations in the frequency spectrum of the displacement at a point on the rotor is studied.

Results and Discussion

Eigenfrequency analysis

The mode shape of the turbocharger for the fourth mode is shown in Figure 2. In this mode primarily the compressor undergoes the whirl of significant amplitude as compared to the other parts of the rotor. Tilt in both the turbine and the compressor is also significant.

Figure 2: Mode shape of the turbocharger.

The whirl plot for the sixth mode is shown in Figure 3. In this mode whirling of both compressor and turbine is significant.

Figure 3: Whirl plot.

Campbell plots, shown in Figure 4 and Figure 5, compares the eigenfrequency variation of the rotor excluding and including the cross-coupled stiffness of the bearings, respectively. There is no appreciable change in the eigenfrequency variation due to cross-coupled stiffness except that third and fourth modes frequencies are more closer in the presence of cross-coupled stiffness.

Figure 4: Campbell plot without cross-coupled stiffness.

Figure 5: Campbell plot with cross-coupled stiffness.

The logarithmic decrement is a parameter that gives the stability state of the system. If the logarithmic decrement is positive the response is said to be stable and vice versa. A zero value indicates no damping in that particular mode. The expression for the logarithmic decrement in terms of eigenvalues is:

A plot of the logarithmic decrement as a function of the rotor angular speed is shown in Figure 6 and Figure 7. This plot shows how the damping in a particular mode changes with the angular speed of the rotor. The logarithmic decrement in the absence of cross-coupled stiffness is positive indicating that the natural modes are stable in the absence of cross-coupled bearing stiffness. In the presence of cross-coupled stiffness, many modes have negative logarithmic decrement even at small rotor speeds. This indicates that the presence of cross-coupled stiffness makes the vibrational modes unstable and hence it is dangerous to operate the turbocharger rotor at these speeds.

Figure 6: Logarithmic decrement without cross-coupled stiffness.

Figure 7: Logarithmic decrement with cross-coupled stiffness.

Cross-coupled stiffness in the hydrodynamic bearings can be reduced by changing the bearing design. For example, the tilted pad bearings are known to have the least cross-coupled stiffness. Other way to control the response is to add more damping in the system, for example, using the squeeze film dampers at various locations.

frequency response analysis

The displacement response with cross-coupled stiffness of the turbocharger operating at 100,000 rpm and subjected to a harmonic loading at 3000 Hz is shown in Figure 8.

Figure 8: Displacement response at 3000 Hz.

The waterfall plot for the displacement at the first bearing location is shown in Figure 9 and Figure 10. The waterfall plot shows the variation in the frequency spectrum of the rotor with the change in its angular speed. In the absence of the cross-coupling forces in the bearing (Figure 9) large peaks are observed for certain combinations of the loading frequency and rotor speed. If the cross-coupling forces are present (Figure 10) peaks are more or less uniform.

Figure 9: Waterfall plot without cross-coupled stiffness.

Figure 10: Waterfall plot with cross-coupled stiffness.

Rotordynamics_Module/Automotive_and_Aerospace/turbocharger_stability_analysis

Modeling Instructions

From the menu, choose .

New

In the window, click

Model Wizard

In the window, click

In the tree, select .

Click .

Click

In the tree, select .

Click

Geometry 1

Import 1 (imp1)

Import the turbocharger geometry.

In the toolbar, click

In the window for , locate the section.

Click

Browse to the model’s Application Libraries folder and double-click the file turbocharger_stability_analysis.mphbin.

Click

Global Definitions

Create the model parameters.

Parameters 1

In the window, under click .

In the window for , locate the section.

In the table, enter the following settings:


Name	Expression	Value	Description
p	0	0	Parameter to include/exclude cross-coupled stiffness
Ow	10000[rpm]	166.67 1/s	Angular speed of the rotor

Add Material

In the toolbar, click

to open the window.

Go to the window.

In the tree, select .

Click the right end of the split button in the window toolbar.

From the menu, choose .

In the toolbar, click

to close the window.

Definitions

Create the selection for the compressor and turbine for later use.

Compressor

In the toolbar, click

Select Domain 2 only.

In the window for , type Compressor in the text field.

Turbine

In the toolbar, click

Select Domain 1 only.

In the window for , type Turbine in the text field.

Solid Rotor (rotsld)

In the window, under click .

In the window for , locate the section.

In the text field, type Ow.

Set the to to save the computation time. A interpolation can be used for better accuracy.

Click to expand the section. From the list, choose .

Linear Elastic Material 1

Add the material damping in the shaft.

In the window, under click .

Damping 1

In the toolbar, click

and choose .

In the window for , locate the section.

In the αdM text field, type 6.04.

In the βdK text field, type 2e-6.

First Support 1

In the window, under click .

Select Points 209 and 210 only.

Second Support 1

In the window, click .

Select Points 251 and 252 only.

Fixed Axial Rotation 1

In the window, under click .

Select Boundaries 6, 7, 134, and 136 only.

Fixed Axial Rotation 2

Right-click and choose .

Select Boundaries 10, 11, 146, and 149 only.

Journal Bearing 1

In the toolbar, click

and choose .

Select Boundaries 85, 86, 138, and 139 only.

In the window for , locate the section.

Specify the vector as


1	x
0	y
0	z

Locate the section. From the list, choose .

In the ku table, enter the following settings:


1e8	4e7*p
-4e7*p	1e8

In the kθ table, enter the following settings:


0	0
0	0

The parameter p is used for enabling and disabling the cross-coupled stiffness.

Journal Bearing 2

Right-click and choose .

In the window for , locate the section.

Click

Select Boundaries 91, 92, 142, and 143 only.

Locate the section. In the ku table, enter the following settings:


1e8	1e7*p
-1e7*p	1e8

In the kθ table, enter the following settings:


0	0
0	0

Rigid Material: Turbine

In the toolbar, click

and choose .

In the window for , locate the section.

From the list, choose .

In the text field, type Rigid Material: Turbine.

Applied Force 1

In the toolbar, click

and choose .

In the window for , locate the section.

Specify the F vector as


0	x
0	y
1e3	z

Rigid Material: Compressor

In the window, right-click and choose .

In the window for , type Rigid Material: Compressor in the text field.

Locate the section. Click

Select Domain 2 only.

Applied Force 1

In the window, expand the node, then click .

In the window for , locate the section.

Specify the F vector as


1e3	x
0	y
0	z

Mesh 1

Swept 1

In the toolbar, click

In the window for , locate the section.

From the list, choose .

Select Domains 3–7 only.

Free Tetrahedral 1

In the toolbar, click

Size 1

In the window, right-click and choose .

In the window for , locate the section.

From the list, choose .

Click

Study 1

Parametric Sweep

In the toolbar, click

In the window for , locate the section.

Click

twice.

In the table, enter the following settings:


Parameter name	Parameter value list	Parameter unit
p (Parameter to include/exclude cross-coupled stiffness)	0 1
Ow (Angular speed of the rotor)	range(0,5000,100000)	rpm

From the list, choose .

Step 1: Eigenfrequency

In the window, click .

In the window for , locate the section.

Select the check box. In the associated text field, type 9.

In the toolbar, click

Results

Mode Shape (rotsld)

The following instructions will generate the mode shape shown in Figure 2.

In the window for , locate the section.

From the list, choose .

Click the

button in the toolbar.

Click the

button in the toolbar.

In the toolbar, click

You can select the different eigenfrequencies from the list to analyze the corresponding mode shapes.

Whirl (rotsld)

The following instructions will generate the whirl plot shown in Figure 3.

In the window, click .

In the window for , locate the section.

From the list, choose .

Whirl 1

In the window, expand the node, then click .

In the window for , locate the section.

In the text field, type 1.

In the text field, type 20.

Click

In the dialog box, select in the tree.

Click .

Click the

button in the toolbar.

In the toolbar, click

Click

The Campbell plots excluding and including the cross coupled stiffness effects are shown in Figure 4 and Figure 5, respectively. Follow the instructions below to reproduce them.

In the toolbar, click

Add Predefined Plot

Go to the window.

In the tree, select .

Click in the window toolbar.

In the toolbar, click

Results

Campbell Plot, Fixed Frame (rotsld)

In the window, under click .

In the window for , locate the section.

From the list, choose .

Click the

button in the toolbar.

In the toolbar, click

Switch the parameter p from to to analyze the effect of the cross-coupled stiffness.

From the list, choose .

In the toolbar, click

Logarithmic Decrement

The following instructions generate the plots shown in Figure 6 and Figure 7.

In the toolbar, click

and choose .

In the window for , type Logarithmic Decrement in the text field.

Locate the section. From the list, choose .

From the list, choose .

Click to expand the section. From the list, choose .

Click the

button in the toolbar.

Global 1

Right-click and choose .

In the window for , click in the upper-right corner of the section. From the menu, choose .

Locate the section. From the list, choose .

From the list, choose .

In the text field, type rotsld.Ovg.

Click to expand the section. Find the subsection. From the list, choose .

From the list, choose .

Find the subsection. From the list, choose .

Click the

button in the toolbar.

In the toolbar, click

Switch the value of the parameter p from to to analyze the effect of cross-coupled stiffness on logarithmic decrement.

Logarithmic Decrement

In the window, click .

In the window for , locate the section.

From the list, choose .

Click the

button in the toolbar.

In the toolbar, click

Animation 1

Finally you can create the animation of the using the following instructions.

In the toolbar, click

and choose .

In the window for , locate the section.

From the list, choose .

Locate the section. From the list, choose .

Click the

button in the toolbar.

Root

is finished now. Now you will analyze the harmonic response of the turbocharger due to external forces on turbine and compressor.

Add Study

In the toolbar, click

to open the window.

Go to the window.

Find the subsection. In the tree, select .

Click in the window toolbar.

In the toolbar, click

to close the window.

Study 2

Step 1: Frequency Domain

In the window for , locate the section.

In the text field, type range(100,100,3000).

In the toolbar, click and choose .

Parametric Sweep

In the toolbar, click

In the window for , locate the section.

Click

twice.

In the table, enter the following settings:


Parameter name	Parameter value list	Parameter unit
p (Parameter to include/exclude cross-coupled stiffness)	0 1
Ow (Angular speed of the rotor)	range(2e3,2e3,1e5)	rpm

From the list, choose .

In the toolbar, click

Results

Displacement (rotsld)

The first default plot for is a stress plot, which is only meaningful in an elastic domain. Change this plot to show the displacement response as in Figure 8 using the following instructions.