PDF
Sound Transmission Loss Through a Concrete Wall
Introduction
This model presents a practical and efficient method to compute the sound transmission loss (STL) through a building component; specifically, this example treats the case of a concrete wall. The method used here is valid as long as the component has little influence on the acoustic field on the source side. The method is based on assuming an ideal diffuse field on the source side and an ideal anechoic termination on the receiver side of the concrete wall. In typical measurement setups, the diffuse sound field is generated in a reverberation room. At low frequencies, the fields that can be obtained are less than perfectly diffuse. The measured STL will therefore to some extent depend on the experimental conditions. From the approach used in this model, you can extract an ideal, experiment-independent STL. The obtained results are compared to published experimental data and show good agreement.
Model Definition
In this tutorial, the sound transmission loss (STL) through a concrete wall is modeled using an approach that is well suited for numerical simulations. A review of STL measurement techniques and theory is given below in order to motivate the simulation approach used here. Following this discussion, the method is described in detail.
Sound Transmission Loss (STL)
The STL through a building component, like a door, a window, a wall segment, or a sound insulation structure, is defined as the ratio expressed in dB of the total incident power Pin on the structure relative to the total transmitted power Ptr:
(1)
The STL is defined for conditions where the acoustic field on the source side is diffuse. Several standards exist for the measurement of the STL, for example, ASTM E90 or ISO 10140. Common to the methods is that they are devised in order to directly or indirectly measure the incident and transmitted power. Typically, a so-called two-room method is used. The two most common configurations both use a reverberation room on the source side. The first also uses a reverberation room on the receiver side (reverberant-reverberant) while the second uses an anechoic room on the receiver side (reverberant-anechoic). The two configurations are sketched in Figure 1.
Figure 1: The two variations of the two-room configuration for measuring the sound transmission loss: (top) both source and receiver reverberation rooms, and (bottom) the source reverberation room and receiver anechoic room.
In both cases, the incident power on the source side is computed as
(2)
where Ss is the area of the test surface on the source side (the area of the concrete wall tested), prms is the RMS pressure in the source room, ρ0 is the air density, and c0 is the speed of sound in air. This expression is derived by considering the incident power on a surface in an ideal diffuse acoustic field; see Ref. 1 and Ref. 2.
The expressions used to compute the incident and transmitted power for the reverberant-reverberant case are only valid as long as the acoustic field is diffuse. A measure for the upper limit of modal behavior is given by the Schroeder frequency
(3)
where V is the room volume and T60 is the reverberation time; see Ref. 1. A room of volume V is said to be acoustically large when the studied frequency f is larger than the Schroeder frequency, giving the condition
(4)
Reverberant-Reverberant Setup
In the setup where the receiver room is a reverberation room (Figure 1 top) and the sound field is assumed diffuse, the transmitted power is given by
(5)
where prms is the RMS pressure in the receiver room and Ar is the receiver room absorption area, that is, the sum of products between each surface area Si and its absorption coefficient αi. The expression stems from an energy balance consideration where the total absorbed energy is equal to the radiated energy of the source. Combining Equation 2 and Equation 5 gives the expression for the STL for the reverberant-reverberant setup
(6)
where SPLs and SPLr are the average sound pressure levels in the source and the receiver room, respectively. Averaging is done on the squared pressure before transforming to the dB scale.
Note that a correction to Equation 5 is sometimes introduced based on the Waterhouse expression. In a room with a diffuse field, the RMS pressure at the walls will be larger by a factor 2 because each incident wave is coherent with its corresponding reflected wave, see Ref. 2. The corrected expression reads
(7)
where EDT is the early decay time, Vr is the receiver room volume, Sr the receiver room surface area, and λ is the wavelength.
Reverberant-Anechoic Setup
In the reverberant-anechoic configuration (Figure 1 bottom), the transmitted power is directly measured on the receiver side using an intensity probe. The measurement is performed in several locations in front of the test element and averaged. The transmitted power is then simply given by
(8)
combining this expression with Equation 1 and Equation 2 gives
(9)
SILtr is the transmitted sound intensity level, and for flat samples Ss = Sr. The numeric constant stems directly from the definitions of SPL and SIL and the equations for the power, it is expressed as
(10)
where pref = 20 µPa, Iref = 10−12 W/m, ρ0 =1.2 kg/m3, and c0 = 343 m/s.
Estimation Model for the STL
The STL for isotropic panels made of an elastic material like the wall studied here has a general frequency dependent behavior that is sketched in Figure 2; see Ref. 3. At low frequencies, below the first mechanical resonance f11 of the structure, the STL is controlled by stiffness. At and around the resonance the STL drops drastically as the structure acts as an optimal transmitter (dips can also occur for the second mode). Above the first resonance the STL becomes controlled by mass. This behavior covers a relatively large frequency band where the STL increases with 6 dB per octave. Then, the STL decreases around the critical frequency fc, in a region called the coincidence region. Coincidence happens when the wavelengths of the pressure waves in the fluid are comparable to the wavelengths of the flexural waves in the structure. Above this region the STL increases. It is first controlled by damping with a 9 dB per octave increase, before it approaches the mass law behavior again.
Figure 2: Schematic representation of the frequency dependency of the STL for isotropic panels.
Several analytical prediction models exist for the STL of simply supported panels, see Ref. 3. One mass law model for 1/3 octave STL values, called Sharp’s equation, is given as
(11)
where m = ρT is the mass per unit area of the structure, ρ is the density of the structure, and T is the thickness of the panel (here the wall, see Figure 4). Note that the predicted STL from Sharp’s equation will in practice exceed the actual STL. This is because the equation assumes an ideal limp panel and does not take into account the panel stiffness. This same trend is seen in the model results discussed below. The slope in the mass law region will obey the 6 dB per octave trend. A doubling of the wall thickness will double the value of m and thus results in a 6 dB increase in STL for a given frequency.
Simulation Model Setup
When simulating the STL it is preferable to avoid modeling the source and receiver rooms as this would be computationally extremely expensive. Instead, the setup is based on assuming an ideal diffuse field on the source side and an ideal anechoic termination on the receiver side of the test sample. The model also assumes that the test sample has little influence on the sound field on the source side. This is true for relatively stiff structures with low acoustic absorption properties. This is the case for the concrete wall studied in this example. The sound field on the source side can then be defined as a sum of 2N uncorrelated plane waves moving in random directions. It can also be assumed that one half of these waves travels in the negative x direction and the other half in the positive x direction. Knowing that the concrete wall is located in the x = 0 plane, only the waves traveling in the positive x direction contribute to the incident pressure on the wall surface. The source room pressure field traveling in the positive x direction is then
(12)
Here the polar angles and , and the phase are independent random numbers. Furthermore, A is the amplitude of the plane waves. and Φn are taken directly from uniform distributions whereas θn is obtained as , with qn being a random variable with uniform distribution between 0 and 1. This ensures a uniform distribution of wave numbers over the desired hemisphere. In the model, a new set of random numbers is generated for each n in the sum. The term ensures that the field has a constant intensity for any choice of N. Because the plane waves are uncorrelated, the total mean square pressure in the source room is , with the term 2px,room accounting for the total diffuse field (positive and negative x directions). The theoretical limit for large N of the mean square pressure in the room (away from walls) is .
The concrete wall is located at x = 0, where the incident diffuse field is reflected. The reflected component of the field is
(13).
The reflected field is coherent with the incident field, as discussed for Equation 5. At the surface of the concrete wall, the total pressure load applied to the structure is the sum of the incident and reflected pressures:
(14)
In the model, the room pressure, the reflected pressure, and wall pressures are defined as variables under Component 1>Definitions.
Figure 3: Model setup of the concrete wall with an ideal diffuse field on the source side and an ideal anechoic termination on the receiver side.
The wall pressure pwall is applied as a load on the source side of the concrete wall. On the receiver side, a perfect anechoic room is modeled using an air domain terminated by a perfectly matched layer (PML). The model setup is sketched in Figure 4.
The concrete wall has a height of H = 4.37 m, a width of W = 2.84 m, and a thickness of T = 203 mm. The density of the concrete is ρ = 2275 kg/m3, its Young’s modulus is E = 31.6 GPa, its Poisson’s ratio is ν = 0.2, and a typical value of 0.01 is used as the isotropic loss factor. The wall size and material data is taken from the test configuration 76–77 described in Ref. 4. The wall is assumed to be fixed at its outer boundary and placed in an ideal surrounding wall that does not contribute to the STL.
Note that the fixed constraint used here is different from the “simply supported” condition (a hinge-like condition) often used in the analytical prediction models. To precisely predict measurements or model the behavior of building components in-situ a good description of the outer boundary conditions is of course required. The condition used will, for example, have a significant influence on the low-frequency stiffness controlled behavior of the STL.
Results and Discussion
The incident intensity distribution on the concrete wall is depicted in Figure 5, evaluated at 125 Hz, 250 Hz, 500 Hz, and 1000 Hz. The distribution is not dependent on the solved model but only on the randomness and number of terms in the expression for the room pressure field px,room from Equation 12.
Figure 4: The incident sound intensity evaluated on the concrete wall surface.
The incident intensity on the test sample is computed using the definition of sound intensity with the incident sound pressure and particle velocity as
(15)
The spatial distribution of the transmitted (radiated) sound intensity is displayed in Figure 6, for the same frequencies as the incident intensity. The transmitted intensity depends on the solved problem and is computed as the total intensity at the concrete surface on the receiver side. It is given as
(16)
where pt is the total acoustic pressure and u is the structural displacement in the x direction. Both variables are solved for in the model.
Figure 5: The transmitted intensity evaluated on the concrete wall surface.
The displacement of the concrete wall as well as the sound pressure in the receiver room are shown in Figure 6 for the same four frequencies. Comparing this result to Figure 6, it is evident that the transmitted intensity field is controlled by the displacement of the structure.
Figure 6: The displacement of the concrete wall and the pressure on the receiver side.
At low frequencies, the displacement distribution is strongly dictated by the possible structural modes shown in Figure 9. For example, the displacement at 125 Hz is closely related to the first mode, while the third mode dominates the displacement at 250 Hz.
Figure 7: The first three modes of the structure.
The sound transmission loss (STL), computed using Equation 1, Equation 2, and Equation 8, is plotted in Figure 10 and Figure 9. The STL is depicted as a continuous line (evaluated for all the computed frequencies) as well as in octave bands or 1/3 octave bands, respectively. Both graphs also include the typically measured STL. The data is adapted from Ref. 4 and shows good agreement.
The two dips found in the STL curve correspond to the first two structural modes seen in Figure 9. They occur at f11 = 113 Hz and f12 = 170 Hz. Below these frequencies the STL is controlled by stiffness and depends highly on the boundary conditions applied to the structure. The mass law behavior applies above these.
In Figure 9 the estimated STL using Sharp’s model from Equation 11 is also plotted in the region where the mass law applies. Despite lower values, the computed STL is seen to follow the slope of the estimate with a 6 dB per octave increase. This is expected as Sharp’s equation assumes a limp structure and does not include the stiffness effects.
Figure 8: Sound transmission loss (STL) through the concrete wall with octave bands.
Figure 9: Sound transmission loss (STL) through the concrete wall with 1/3 octave bands.
Finally, three different methods to compute the incident power on the wall are compared in Figure 7. The blue graph shows the value given by the surface integral of Equation 15. The green and red graphs represent the values given by Equation 2 where the RMS pressures are calculated respectively from the source room sound field and from the theoretical diffuse field limit. The large fluctuations at low frequencies, where the wavelength is comparable to the wall size, indicate that the diffuse sound field assumption does not hold. In measurement conditions, the sound field would also not be diffuse at these low frequencies.
Figure 10: The incident power on the wall evaluated with three different methods.
Notes About the COMSOL Implementation
The model is based on several assumptions:
•
The sound field in the source room is perfectly diffuse.
•
The acoustic behavior in the receiver room is perfectly anechoic.
•
The test sample under analysis has a negligible influence on the sound field in the source room.
If the test sample does not meet the last requirement, the acoustics of the source room needs to be modeled and coupled with the structure in order to compute the sound transmission loss.
References
1. H. Kuttruff, Room Acoustics, CRC Press, Fifth Edition, 2009.
2. F. Jacobsen, “The Sound Field in a Reverberation Room,” Lecture Note no. 31261, Acoustic, Technology, Technical University of Denmark, 2011.
3. D.A. Bies, C. Hansen, and C. Howard, “Engineering Noise Control,” 5th Edition, CRC Press, 2017.
4. A. Litvin and H.W. Belliston, “Sound Transmission Loss Through Concrete and Concrete Masonry Walls,” American Concrete Institute, Journal Proceedings, vol. 45, pp. 641–646, 1978.
Application Library path: Acoustics_Module/Building_and_Room_Acoustics/sound_transmission_loss_concrete
Modeling Instructions
From the File menu, choose New.
New
In the New window, click  Model Wizard.
Model Wizard
1
In the Model Wizard window, click  3D.
2
In the Select Physics tree, select Acoustics>Acoustic-Structure Interaction>Acoustic-Solid Interaction, Frequency Domain.
3
Click Add.
4
Click  Study.
5
In the Select Study tree, select General Studies>Frequency Domain.
6
Click  Done.
Global Definitions
Parameters 1
1
In the Model Builder window, under Global Definitions click Parameters 1.
2
In the Settings window for Parameters, locate the Parameters section.
3
Click  Load from File.
4
Browse to the model’s Application Libraries folder and double-click the file sound_transmission_loss_concrete_parameters.txt.
Create an interpolation function that contains typical measurement data of the STL for the concrete wall.
Interpolation 1 (int1)
1
In the Home toolbar, click  Functions and choose Global>Interpolation.
2
In the Settings window for Interpolation, locate the Definition section.
3
From the Data source list, choose File.
4
Click  Browse.
5
Browse to the model’s Application Libraries folder and double-click the file sound_transmission_loss_concrete_measurement_data.txt.
6
Find the Functions subsection. In the table, enter the following settings:
Function name
Position in file
STL_typical
1
7
Click  Import.
8
Locate the Interpolation and Extrapolation section. From the Interpolation list, choose Piecewise cubic.
9
Locate the Units section. In the Argument table, enter the following settings:
Argument
Unit
t
Hz
10
In the Function table, enter the following settings:
Function
Unit
STL_typical
dB
Geometry 1
Block 1 (blk1)
1
In the Geometry toolbar, click  Block.
2
In the Settings window for Block, locate the Size and Shape section.
3
In the Width text field, type T.
4
In the Depth text field, type W.
5
In the Height text field, type H.
Block 2 (blk2)
1
In the Geometry toolbar, click  Block.
2
In the Settings window for Block, locate the Size and Shape section.
3
In the Width text field, type 3*T.
4
In the Depth text field, type W+4*T.
5
In the Height text field, type H+4*T.
6
Locate the Position section. In the x text field, type T.
7
In the y text field, type -2*T.
8
In the z text field, type -2*T.
9
Click to expand the Layers section. In the table, enter the following settings:
Layer name
Thickness (m)
Layer 1
T
10
Find the Layer position subsection. Select the Right check box.
11
Select the Front check box.
12
Select the Back check box.
13
Select the Top check box.
14
Click  Build All Objects.
Pressure Acoustics, Frequency Domain (acpr)
1
In the Model Builder window, under Component 1 (comp1) click Pressure Acoustics, Frequency Domain (acpr).
2
Select Domains 2–19 only.
Solid Mechanics (solid)
1
In the Model Builder window, under Component 1 (comp1) click Solid Mechanics (solid).
2
In the Settings window for Solid Mechanics, locate the Domain Selection section.
3
Click  Clear Selection.
4
Select Domain 1 only.
Multiphysics
Acoustic-Structure Boundary 1 (asb1)
Click the  Wireframe Rendering button in the Graphics toolbar.
Add Material
1
In the Home toolbar, click  Add Material to open the Add Material window.
2
Go to the Add Material window.
3
In the tree, select Built-in>Air.
4
Click Add to Component in the window toolbar.
5
In the Home toolbar, click  Add Material to close the Add Material window.
Materials
Concrete
1
In the Model Builder window, under Component 1 (comp1) right-click Materials and choose Blank Material.
2
In the Settings window for Material, type Concrete in the Label text field.
3
Select Domain 1 only.
4
Locate the Material Contents section. In the table, enter the following settings:
Property
Variable
Value
Unit
Property group
Young’s modulus
E
31.6e9
Pa
Young’s modulus and Poisson’s ratio
Poisson’s ratio
nu
0.2
1
Young’s modulus and Poisson’s ratio
Density
rho
2275
kg/m³
Basic
Definitions
Variables: Diffuse Field
1
In the Model Builder window, under Component 1 (comp1) right-click Definitions and choose Variables.
2
In the Settings window for Variables, type Variables: Diffuse Field in the Label text field.
3
Locate the Variables section. Click  Load from File.
4
Browse to the model’s Application Libraries folder and double-click the file sound_transmission_loss_concrete_variables_diffuse.txt.
Variables: STL
1
In the Model Builder window, right-click Definitions and choose Variables.
2
In the Settings window for Variables, type Variables: STL in the Label text field.
3
Locate the Variables section. Click  Load from File.
4
Browse to the model’s Application Libraries folder and double-click the file sound_transmission_loss_concrete_variables_stl.txt.
Random 1 (rn1)
1
In the Home toolbar, click  Functions and choose Local>Random.
2
In the Settings window for Random, type costheta_rnd in the Function name text field.
3
Locate the Parameters section. In the Number of arguments text field, type 4.
4
In the Mean text field, type 0.5.
Random 2 (rn2)
1
In the Home toolbar, click  Functions and choose Local>Random.
2
In the Settings window for Random, type phi_rnd in the Function name text field.
3
Locate the Parameters section. In the Number of arguments text field, type 4.
4
In the Mean text field, type pi.
5
In the Range text field, type 2*pi.
Random 3 (rn3)
1
In the Home toolbar, click  Functions and choose Local>Random.
2
In the Settings window for Random, type phase_rnd in the Function name text field.
3
Locate the Parameters section. In the Number of arguments text field, type 4.
4
In the Mean text field, type pi.
5
In the Range text field, type 2*pi.
Integration 1 (intop1)
1
In the Definitions toolbar, click  Nonlocal Couplings and choose Integration.
2
In the Settings window for Integration, type intop_in in the Operator name text field.
3
Locate the Source Selection section. From the Geometric entity level list, choose Boundary.
4
Select Boundary 1 only.
Integration 2 (intop2)
1
In the Definitions toolbar, click  Nonlocal Couplings and choose Integration.
2
In the Settings window for Integration, type intop_tr in the Operator name text field.
3
Locate the Source Selection section. From the Geometric entity level list, choose Boundary.
4
Select Boundary 26 only.
Save solution on boundaries
1
In the Definitions toolbar, click  Explicit.
2
In the Settings window for Explicit, type Save solution on boundaries in the Label text field.
3
Locate the Input Entities section. From the Geometric entity level list, choose Boundary.
4
Select Boundaries 1–5, 19, 26, and 53 only.
Perfectly Matched Layer 1 (pml1)
1
In the Definitions toolbar, click  Perfectly Matched Layer.
2
Select Domains 2–5 and 7–19 only.
3
In the Settings window for Perfectly Matched Layer, locate the Scaling section.
4
In the PML scaling curvature parameter text field, type 3.
Solid Mechanics (solid)
Linear Elastic Material 1
In the Model Builder window, under Component 1 (comp1)>Solid Mechanics (solid) click Linear Elastic Material 1.
Damping 1
1
In the Physics toolbar, click  Attributes and choose Damping.
2
In the Settings window for Damping, locate the Damping Settings section.
3
From the Damping type list, choose Isotropic loss factor.
Remember to go back to the Concrete material and add the value for the isotropic loss factor.
Materials
Concrete (mat2)
1
In the Model Builder window, under Component 1 (comp1)>Materials click Concrete (mat2).
2
In the Settings window for Material, locate the Material Contents section.
3
In the table, enter the following settings:
Property
Variable
Value
Unit
Property group
Isotropic structural loss factor
eta_s
0.01
1
Basic
Solid Mechanics (solid)
Fixed Constraint 1
1
In the Physics toolbar, click  Boundaries and choose Fixed Constraint.
2
Select Boundaries 2–5 only.
The selection should look like this.
Boundary Load 1
1
In the Physics toolbar, click  Boundaries and choose Boundary Load.
2
Select Boundary 1 only.
3
In the Settings window for Boundary Load, locate the Force section.
4
From the Load type list, choose Pressure.
5
In the p text field, type p_wall.
In this model, the mesh is set up manually. Proceed by directly adding the desired mesh component. The following steps show how to create a swept mesh to reduce the computation time.
Mesh 1
Free Quad 1
1
In the Mesh toolbar, click  Boundary and choose Free Quad.
2
Select Boundary 19 only.
Size
1
In the Model Builder window, click Size.
2
In the Settings window for Size, locate the Element Size section.
3
Click the Custom button.
4
Locate the Element Size Parameters section. In the Maximum element size text field, type c0/fmax/5.
5
In the Minimum element size text field, type c0/fmax/6.
Mapped 1
1
In the Mesh toolbar, click  Boundary and choose Mapped.
2
Select Boundaries 6, 9, 12, 16, 22, 26, 27, 30, and 33 only.
Distribution 1
1
Right-click Mapped 1 and choose Distribution.
2
Select Edges 16, 26, 35, and 39 only.
3
In the Settings window for Distribution, locate the Distribution section.
4
In the Number of elements text field, type 8.
5
Click  Build All.
Swept 1
In the Mesh toolbar, click  Swept.
Distribution 1
1
Right-click Swept 1 and choose Distribution.
2
In the Settings window for Distribution, locate the Domain Selection section.
3
Click  Clear Selection.
4
Select Domain 1 only.
5
Locate the Distribution section. In the Number of elements text field, type 2.
Distribution 2
1
In the Model Builder window, right-click Swept 1 and choose Distribution.
2
In the Settings window for Distribution, locate the Domain Selection section.
3
Click  Clear Selection.
4
In the Graphics window toolbar, clicknext to  Select Box, then choose Entity Intersects.
5
Select Domains 11–19 only.
6
Locate the Distribution section. In the Number of elements text field, type 8.
7
Click  Build All.
The mesh should look like this.
Study 1
Solution 1 (sol1)
1
In the Study toolbar, click  Show Default Solver.
The default solver works, but to reduce the computation time, enable the second suggested iterative solver. This solver is both faster and more memory efficient than the default direct solver. It uses a multigrid preconditioner for the acoustic variables and a direct preconditioner for the solid mechanics variables.
Step 1: Frequency Domain
1
In the Model Builder window, under Study 1 click Step 1: Frequency Domain.
2
In the Settings window for Frequency Domain, locate the Study Settings section.
3
From the Reuse solution from previous step list, choose No.
Solution 1 (sol1)
1
In the Model Builder window, expand the Solution 1 (sol1) node.
2
In the Model Builder window, expand the Study 1>Solver Configurations>Solution 1 (sol1)>Stationary Solver 1 node.
3
Right-click Study 1>Solver Configurations>Solution 1 (sol1)>Stationary Solver 1>Suggested Iterative Solver (GMRES with GMG and Direct Precond.) (asb1) and choose Enable.
Step 1: Frequency Domain
1
In the Model Builder window, under Study 1 click Step 1: Frequency Domain.
2
In the Settings window for Frequency Domain, locate the Study Settings section.
3
Click  Range.
4
In the Range dialog box, choose ISO preferred frequencies from the Entry method list.
5
In the Start frequency text field, type 35.
6
In the Stop frequency text field, type fmax.
7
From the Interval list, choose 1/12 octave.
8
Click Replace.
9
In the Settings window for Frequency Domain, click to expand the Values of Dependent Variables section.
10
Find the Store fields in output subsection. From the Settings list, choose For selections.
11
Under Selections, click  Add.
To reduce the model size when saved, only store the solution on the selected boundaries.
12
In the Add dialog box, select Save solution on boundaries in the Selections list.
13
Click OK.
14
In the Study toolbar, click  Compute.
Results
Acoustic Pressure (acpr)
Inspect the default plots generated, you can change the evaluation frequency if needed. Notice that the isosurface plot is less interesting as we have only stored the solution on boundaries.
Sound Pressure Level (acpr)
Acoustic Pressure, Isosurfaces (acpr)
Stress (solid)
1
In the Model Builder window, click Stress (solid).
2
In the Settings window for 3D Plot Group, locate the Color Legend section.
3
Select the Show units check box.
Next, create plots of the incident and transmitted intensity, the displacement, as well as 1D plots of the STL.
Incident Intensity
1
In the Home toolbar, click  Add Plot Group and choose 3D Plot Group.
2
In the Settings window for 3D Plot Group, type Incident Intensity in the Label text field.
3
Locate the Color Legend section. Select the Show units check box.
Surface 1
1
Right-click Incident Intensity and choose Surface.
2
In the Settings window for Surface, locate the Expression section.
3
In the Expression text field, type Ix_room.
Selection 1
1
Right-click Surface 1 and choose Selection.
2
Select Boundary 1 only.
3
In the Incident Intensity toolbar, click  Plot.
The plot is depicted at four frequencies in Figure 4.
Transmitted Intensity
1
In the Home toolbar, click  Add Plot Group and choose 3D Plot Group.
2
In the Settings window for 3D Plot Group, type Transmitted Intensity in the Label text field.
3
Locate the Color Legend section. Select the Show units check box.
Surface 1
1
Right-click Transmitted Intensity and choose Surface.
2
In the Settings window for Surface, locate the Expression section.
3
In the Expression text field, type acpr.Ix.
Selection 1
1
Right-click Surface 1 and choose Selection.
2
Select Boundary 26 only.
3
In the Transmitted Intensity toolbar, click  Plot.
The plot is depicted at four frequencies in Figure 5.
4
In the Home toolbar, click  Windows and choose Add Predefined Plot.
Add Predefined Plot
1
Go to the Add Predefined Plot window.
2
In the tree, select Study 1/Solution 1 (sol1)>Solid Mechanics>Displacement (solid).
3
Click Add Plot in the window toolbar.
4
In the Home toolbar, click  Add Predefined Plot.
Results
Displacement (solid)
1
In the Model Builder window, under Results click Displacement (solid).
2
In the Settings window for 3D Plot Group, click to expand the Title section.
3
From the Title type list, choose Manual.
4
In the Title text area, type f = eval(freq) Hz.
5
Locate the Plot Settings section. Clear the Plot dataset edges check box.
6
Locate the Color Legend section. Select the Show units check box.
Volume 1
1
In the Model Builder window, expand the Displacement (solid) node.
2
Right-click Volume 1 and choose Disable.
Surface 1
1
In the Model Builder window, right-click Displacement (solid) and choose Surface.
2
In the Settings window for Surface, locate the Expression section.
3
In the Expression text field, type solid.disp.
Deformation 1
Right-click Surface 1 and choose Deformation.
Filter 1
1
In the Model Builder window, right-click Surface 1 and choose Filter.
2
In the Settings window for Filter, locate the Element Selection section.
3
In the Logical expression for inclusion text field, type z>1.5[m].
Surface 2
1
In the Model Builder window, right-click Displacement (solid) and choose Surface.
2
In the Settings window for Surface, locate the Coloring and Style section.
3
Click  Change Color Table.
4
In the Color Table dialog box, select Wave>Wave in the tree.
5
Click OK.
6
In the Settings window for Surface, locate the Coloring and Style section.
7
From the Scale list, choose Linear symmetric.
Selection 1
1
Right-click Surface 2 and choose Selection.
2
Select Boundary 53 only.
3
In the Displacement (solid) toolbar, click  Plot.
The plot is depicted at four frequencies in Figure 6.
Postprocessing the STL variables is time consuming, so in order to save time setting up the next three plots (avoiding automatic plotting when formatting the plots), enable the Only plot when requested option.
4
In the Model Builder window, click Results.
5
In the Settings window for Results, locate the Update of Results section.
6
Select the Only plot when requested check box.
STL: P_in/P_tr (octaves)
1
In the Home toolbar, click  Add Plot Group and choose 1D Plot Group.
2
In the Settings window for 1D Plot Group, type STL: P_in/P_tr (octaves) in the Label text field.
3
Click to expand the Title section. From the Title type list, choose Manual.
4
In the Title text area, type Sound Transmission Loss (octaves).
5
Locate the Plot Settings section.
6
Select the x-axis label check box. In the associated text field, type f (Hz).
7
Select the y-axis label check box. In the associated text field, type STL (dB).
8
Locate the Legend section. From the Position list, choose Lower left.
Octave Band 1
1
In the STL: P_in/P_tr (octaves) toolbar, click  More Plots and choose Octave Band.
2
In the Settings window for Octave Band, locate the Selection section.
3
From the Geometric entity level list, choose Global.
4
Locate the y-Axis Data section. From the Expression type list, choose Power.
5
In the Expression text field, type P_in.
6
In the Power reference text field, type P_tr.
7
Locate the Plot section. From the Quantity list, choose Continuous power spectral density.
Octave Band 2
1
Right-click Octave Band 1 and choose Duplicate.
2
In the Settings window for Octave Band, locate the Plot section.
3
From the Quantity list, choose Band average power spectral density.
4
Click to expand the Coloring and Style section. From the Type list, choose Outline.
Global 1
1
In the Model Builder window, right-click STL: P_in/P_tr (octaves) and choose Global.
2
In the Settings window for Global, locate the Data section.
3
From the Dataset list, choose Study 1/Solution 1 (sol1).
4
From the Parameter selection (freq) list, choose From list.
5
From the Parameter values list select the frequencies from 100 Hz to 1180 Hz, where the measurements are valid.
6
Locate the y-Axis Data section. In the table, enter the following settings:
Expression
Unit
Description
STL_typical(freq)
 
Typical Measurements
7
In the STL: P_in/P_tr (octaves) toolbar, click  Plot.
The STL plot, with the octave evaluation, is depicted in Figure 8.
STL: P_in/P_tr (1/3 octaves)
1
Right-click STL: P_in/P_tr (octaves) and choose Duplicate.
2
In the Settings window for 1D Plot Group, type STL: P_in/P_tr (1/3 octaves) in the Label text field.
3
Locate the Title section. In the Title text area, type Sound Transmission Loss (1/3 octaves).
Octave Band 2
1
In the Model Builder window, expand the STL: P_in/P_tr (1/3 octaves) node, then click Octave Band 2.
2
In the Settings window for Octave Band, locate the Plot section.
3
From the Band type list, choose 1/3 octave.
Global 2
1
In the Model Builder window, right-click STL: P_in/P_tr (1/3 octaves) and choose Global.
2
In the Settings window for Global, locate the Data section.
3
From the Dataset list, choose Study 1/Solution 1 (sol1).
4
From the Parameter selection (freq) list, choose From list.
5
From the Parameter values list select the frequencies from 200 Hz to 1180 Hz, to plot Sharp’s equation here.
6
Locate the y-Axis Data section. In the table, enter the following settings:
Expression
Unit
Description
10*log10(1+(pi*freq*m/(rho0*c0))^2)-5.5
 
Sharp's Equation (mass law)
7
In the STL: P_in/P_tr (1/3 octaves) toolbar, click  Plot.
The STL plot, with the 1/3 octave evaluation and Sharp’s equation, is depicted in Figure 9.
Incident Power (three methods)
1
In the Home toolbar, click  Add Plot Group and choose 1D Plot Group.
2
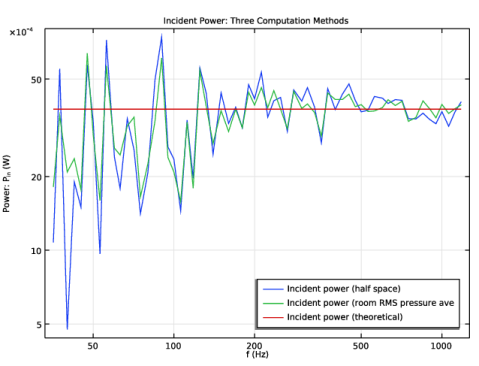
In the Settings window for 1D Plot Group, type Incident Power (three methods) in the Label text field.
3
Click to collapse the Title section. Click to expand the Title section. From the Title type list, choose Manual.
4
In the Title text area, type Incident Power: Three Computation Methods.
5
Locate the Plot Settings section.
6
Select the x-axis label check box. In the associated text field, type f (Hz).
7
Select the y-axis label check box. In the associated text field, type Power: P<sub>in</sub> (W).
8
Locate the Axis section. Select the x-axis log scale check box.
9
Select the y-axis log scale check box.
10
Locate the Legend section. From the Position list, choose Lower right.
Global 1
1
Right-click Incident Power (three methods) and choose Global.
2
In the Settings window for Global, locate the y-Axis Data section.
3
In the table, enter the following settings:
Expression
Unit
Description
P_in
W
Incident power (half space)
P_in_proom
W
Incident power (room RMS pressure average)
P_in_theo
W
Incident power (theoretical)
4
In the Incident Power (three methods) toolbar, click  Plot.
The power plot is depicted in Figure 10.
Proceed and add a second study to perform an eigenfrequency analysis of the structure (the wall). When adding the study de-select the acoustic and the multiphysics coupling. In the analysis setup look for the first 3 modes.
Add Study
1
In the Home toolbar, click  Add Study to open the Add Study window.
2
Go to the Add Study window.
3
Find the Studies subsection. In the Select Study tree, select General Studies>Eigenfrequency.
4
Find the Physics interfaces in study subsection. In the table, clear the Solve check box for Pressure Acoustics, Frequency Domain (acpr).
5
Find the Multiphysics couplings in study subsection. In the table, clear the Solve check box for Acoustic-Structure Boundary 1 (asb1).
6
Click Add Study in the window toolbar.
7
In the Home toolbar, click  Add Study to close the Add Study window.
Study 2
Step 1: Eigenfrequency
1
In the Settings window for Eigenfrequency, locate the Study Settings section.
2
Select the Desired number of eigenfrequencies check box. In the associated text field, type 3.
3
From the Eigenfrequency search method around shift list, choose Larger real part.
4
In the Home toolbar, click  Compute.
Results
Mode Shape (solid)
The first three structural modes are depicted in Figure 7. A table with the eigenvalues is also automatically generated. To see the table select the Eigenfrequencies (Study 2) evaluation group node.
Finally, disable the Only plot when requested option for the results. Turn On the option to Save plot data in order to avoid rerendering the STL curves once the model is opened again.
1
In the Model Builder window, click Results.
2
In the Settings window for Results, locate the Update of Results section.
3
Clear the Only plot when requested check box.
4
Locate the Save Data in the Model section. From the Save plot data list, choose On.