where (adjBi)
jk is the
jkth component of the adjoint of the matrix
Bi.
For low-density gas mixtures, the multicomponent Maxwell–Stefan diffusivities, Dij, can be replaced with the binary diffusivities for the species pairs that are present.
Solving for Equation 3-51 leads to a number of algebraic expressions for each of the components in the multicomponent Fick diffusivity matrix. For two- and three-component systems, these are implemented and solved directly by COMSOL Multiphysics. For instance, the component
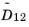
in a ternary system is given by:
where ij are indices in the matrices
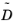
and
N, and ranges from 1 to the number of species,
Q.
where P−1 is the inverse of a matrix
P defined as
The term g in
Equation 3-52 is a scalar value that provides numerical stability and should be of the same order of magnitude as the multicomponent Maxwell–Stefan diffusion coefficients. The physics interface therefore defines
q as the sum of the multicomponent Maxwell–Stefan diffusion coefficients:
Because the multicomponent Fick diffusivity matrix is symmetric, it is sufficient to apply Equation 3-53 to the upper triangle elements of
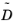
. The remaining elements are obtained by swapping the indices in the matrix.
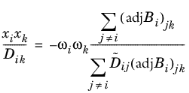 ,
,