Detached Eddy Simulation combines Large Eddy Simulation and RANS, resolving the large turbulent eddies in the bulk and modeling the unresolved eddies, in the bulk using a LES approach, and in the near-wall region using a RANS approach. As for LES, the simulations must be three-dimensional and time dependent. The current implementation of the DES interfaces is suitable for incompressible flow only. Turbulent stresses, modeled by a Boussinesq closure, are added to the existing LES models using the Spalart-Allmaras model (see The Turbulent Flow, Spalart–Allmaras Interface), and the wall distance in the transport equation for
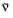
is limited by the size of the mesh cell. The implementation, based on the IDDES method (see
Ref. 1), has been adjusted to comply with residual-based LES models.
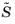
, in the Spalart-Allmaras equation (
Equation 3-153), is modified as
where Dw is the distance to the closest wall and
h is the length of the longest edge of the mesh element. The function
fd is defined as
fstep is 1 for
Dw<0.5275
CDESh and sharply approaches 0 away from the wall. At
Dw=CDESh,
fstep=0.01266. The function
frestore is only non-zero in the region 0
<Dw<0.5275
CDESh, and is added to amplify the RANS length scale, and thereby the turbulent viscosity, outside the viscous sublayer and the log layer. It is defined as
The amplification factor Ψ is purely empirical and is expressed in terms of the Spalart-Allmaras parameters
Inspecting Equation 3-226 and
Equation 3-227, it can be seen that for
Dw<0.5275
CDESh, the DES model operates in RANS mode with an amplified turbulent viscosity within regions outside the viscous sublayer and the log layer (determined by the function
famp). For
Dw>0.5275
CDESh, the DES model operates in DDES (Delayed Detached Eddy Simulation) mode inside attached turbulent boundary layers (determined by the first argument in
Equation 3-227), or smoothly approaches the LES mode of operation away from the wall. The extent of the inner, “protected” RANS region can be controlled by changing the value of the
CDES parameter.