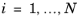
The model equations that are solved in the Phase Transport Mixture Model interfaces are based on the mass conservation of each phase and on conservation of momentum. The mass conservation equation for each (immiscible) phase is given by:

where ρi denotes the density (SI unit: kg/m3), si denotes the volume fraction (dimensionless), and ui denotes the velocity vector (SI unit: m/s) of phase i. In addition, the term Qi denotes a mass source for phase i (SI unit: kg/(m3·s)). It is assumed that the sum of the volume fractions of the phases equals 1:

This means that N − 1 phase volume fractions are independent and are possible to solve for using Equation 6-120. The volume constraint Equation 6-121 is used to reduce the number of dependent variables: one volume fraction, let us say of phase ic (to be specified in the main node of the coupled Phase Transport interface), is expressed using the other volume fractions:

In the Mixture Model multiphysics coupling feature, it is assumed that the phase ic is the continuous phase.

Furthermore, the mass averaged mixture velocity um is defined as:

Here ρ is the mixture density (SI unit: kg/m3) given by:

The velocity ui of phase i is defined as:

where the slip velocity uslip,i (SI unit: m/s) is given by one of the slip velocity models discussed below, and where the last term accounts for the turbulent effects, with Dmd (SI unit: m2/s) a turbulent dispersion coefficient given by

where σT is the turbulent particle Schmidt number (dimensionless). The particle Schmidt number is usually suggested a value ranging from 0.35 to 0.7.

Using the expression above for the velocity ui of phase i, the conservation equation for the dispersed phases can be written as

Here the velocity field ur is the mass average of all slip velocities:

With the previous definitions, the velocity of the continuous phase 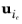can be written in terms of the mixture velocity um and the slip velocities uslip,i as follows:

Here τGm is the sum of the viscous and turbulent stresses (SI unit: kg/(m·s2)). The last term between parenthesis on the first line of Equation 6-130 is called the diffusion stress.

When the Low dispersed phase concentration check box is selected (see The Phase Transport Mixture Model Interfaces), the momentum equation, Equation 6-130, and continuity equation, Equation 6-125, are replaced by

When the Include bubble-induced turbulence check box is selected, a source term Sk, which accounts for extra production of turbulence due to relative motion between the gas bubbles and the liquid, is added to the transport equation for the turbulent kinetic energy, k. This term is given by

In addition, the transport equation for the turbulent energy’s dissipation rate, ε, includes the following source term:

When, on the other hand, the coupled turbulence model includes the specific dissipation rate, ω, the following source term is added to the transport equation for ω:

Suitable values for the model parameters Ck, Cε, and αω are not as well established as the parameters for single-phase flow. In the literature, values within the ranges 0.01 < Ck < 1, 1 < Cε < 1.92 have been suggested (see also Theory for the Bubbly Flow Interfaces), and αω can be defined as .
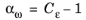