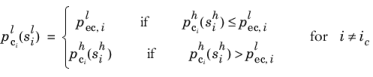Over a boundary between a more permeable and a less permeable porous domain, the macroscopic saturation of the different phases is often discontinuous. The Porous Medium Discontinuity boundary condition implements the following condition on such an interior boundary:

where the superscript l is used to indicate the saturation and capillary pressure on the low permeable side of the boundary, and the superscript h indicates the high permeable side. The first case implements the condition that if the capillary pressure on the more permeable side is lower than the entry capillary pressure on the less permeable side, then the saturation of phase i on the less permeable side equals 0 (or the residual saturation), and the second case implements the condition that if the capillary pressure on the more permeable side is higher than the entry capillary pressure on the less permeable side, the phase i is present on both sides of the interface, and that in this case the capillary pressure is continuous over the interface.

The additional conditions are continuity of the fluxes for all phases and continuity of the pressure of the phase ic computed from the volume constraint. Note that this last condition assumes that this phase is present on both sides of the porous medium discontinuity boundary. In addition, this boundary condition assumes that the phase ic computed from the volume constraint is the wetting phase and it is necessary that the settings for the van Genuchten or Brooks and Corey capillary pressure model match this assumption.

When the automatic settings are used for the Porous Medium Discontinuity boundary condition and if in one or both of the adjacent domains none of the predefined capillary pressure models is selected (van Genuchten or Brooks and Corey), the entry capillary pressure in that domain is determined by substituting 0 for saturation of the phase which is not computed from the volume constraint into the user defined expression for the capillary pressure.

Also note that if in one or both of the adjacent domains the Capillary diffusion model is selected, the Porous Medium Discontinuity boundary condition is not applicable as the entry capillary pressure in this case is not known on both sides of the boundary.