The Reynolds Equation
The equations of fluid flow in thin films are usually formulated on a reference surface in the Eulerian frame. Consider a cylinder, fixed with respect to a stationary reference surface, as shown in Figure 9-3.
Figure 9-3: Reference cylinder, fixed with respect to the stationary reference surface, in a small gap between two surfaces (the wall and the base). The cylinder has outward normal, nc. Its area, projected onto the reference surface, is dA.
The cylinder is fixed with respect to the reference surface, but its height can change due to changes in the position of the base and the wall. Considering the flow in the reference plane, the rate at which mass accumulates within the reference cylinder is determined by the divergence of the mass flow field in the reference plane:
(9-1)
where h=hw+hb and the tangential velocity, vav represents the mean velocity of the flow in the reference plane. Particular care must be taken with respect to the definition of h. The above equation applies if h is measured with respect to a fixed point on the reference surface as a function of time. The reference surface itself must be fixed in space and obviously cannot deform as time progresses. Equations represented on the reference surface are described as Eulerian, that is they are defined in a frame that is fixed with respect to the motion of the fluid or of the body. Fluid flow problems are usually formulated in the Eulerian frame, and COMSOL Multiphysics adopts this convention in most of its fluid flow interfaces. It is useful to note that the Eulerian frame is usually called the spatial frame within the COMSOL Multiphysics interface. When a structure deforms in COMSOL Multiphysics, the spatial frame changes shape.
The wall and the base can, and often do, move with respect to the reference surface. The wall and base are usually the surfaces of mechanical components which are deforming as a result of the pressure building up within the region of fluid flow. When describing the physics of a deforming solid, it is often convenient to work in the Lagrangian frame, which is fixed with respect to a small control volume of the solid. As the solid deforms, the Lagrangian frame moves along with the material contained within the control volume. Using the Lagrangian frame for describing structural deformation means that changes in the local density and material orientation as a result of the distortion of the control volume do not need to be accounted for by complicated transformations. In COMSOL Multiphysics, the Lagrangian frame, usually referred to as the material frame, is used for describing structural deformations. When a structure deforms in COMSOL Multiphysics, the material frame remains in the original configuration of the structure, and the deformation is accounted for by the underlying equations.
Because of the mixed Eulerian-Lagrangian approach adopted within COMSOL Multiphysics, particular care must be taken with the formulation of the Reynolds equations. Typically it is not desirable to represent the geometry of the thin film itself directly, because it is often much smaller than the other components in the model. The equations apply on a single surface in the model, the reference surface. Since we do not want the reference surface to deform, the equations are added in the material frame (even though this frame is normally used in a Lagrangian approach) and manual transformations are added to the system to account for the fact that the structural equations also exist in the material frame, and employ a truly Lagrangian approach.
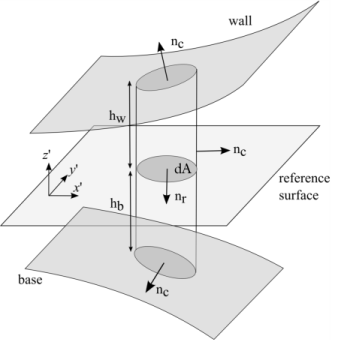
The situation encountered when both the wall and the base undergo a displacement is depicted in Figure 9-4. The Eulerian wall height changes from an initial value hw1 to a final value hw. Similarly the base height changes from an initial value hb1 to hb.
Figure 9-4: Diagram showing the displacement of the wall and the base with respect to the reference surface, and the corresponding change in the height of the channel.
From the figure it is clear that:
(9-2)
It should be noted that similar corrections should be applied to the velocity of the wall, if it is computed from the structural displacement. That is the velocity should be modified by a term proportional to its spatial gradient. This correction term, is however, a second order term, and consequently it is usually negligible in practical circumstances. It is neglected in the thin film flow interface.
Consequently, provided that the definitions of the wall and base height from Equation 9-2 are used, the Reynolds equation takes the form:
(9-3)
The definition of the average flow velocity, vav, together with the forces that act on the structure, remain to be specified.