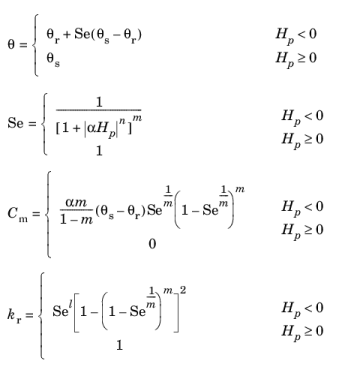
Richards’ equation appears deceptively similar to the saturated flow equation set out in the Darcy’s Law interface, but it is notoriously nonlinear (Ref. 3). Nonlinearities arise because the material and hydraulic properties
θ,
Se,
Cm, and
kr vary for unsaturated conditions (for example, negative pressure) and reach a constant value at saturation (for example, pressure of zero or above). The volume of liquid per porous medium volume,
θ, ranges from a small residual value
θr to the total porosity
θs. Its value is given in a constitutive relation in the model. The effective saturation,
Se, amounts to
θ normalized to a maximum value of 1. The specific moisture capacity,
Cm, describes the change in
θ as the solution progresses, the slope on a plot of
θ versus pressure (or pressure head). The relative permeability,
kr, increases with moisture content and varies from a nominal value to its maximum value at saturation, which reveals that the fluid moves more readily when the porous medium is fully wet.
The analytic formulas of van Genuchten (Ref. 4) and Brooks and Corey (
Ref. 5) are so frequently used that they are synonymous with this variably saturated flow modeling. Posed in terms of pressure head
Hp = p/(ρ g), the analytic expressions require data for the saturated
θs and residual
θr liquid volume fractions as well as constants
α,
n,
m, and
l, which specify a particular medium type.
here, the constitutive parameter m is equal to
1 − 1 / n. With the Brooks and Corey approach, an air-entry pressure distinguishes saturated (
Hp > −1
/α) and unsaturated (
Hp < −1
/α) flow so that
Here, the constitutive parameter m is equal to
1 − 1 / n. COMSOL Multiphysics also provides user-defined options for those who want to incorporate experimental data or arbitrary expressions to define these relationships.