Ideal Cloak

Ideal Cloak¹

Electromagnetic or optical invisibility can be achieved by coating an object with a transparent gradient-index structure that bends the rays of light around the concealed object (Ref. 1). The structure has to be able to do so with radiation incident from any direction, which can be achieved by making it rotationally invariant. Inside this transparent shell, situated is a nontransparent object whose scattering properties in free space are inessential as long as the cloak operates perfectly by preventing any light rays from hitting the object. The concept of invisibility based on omnidirectional cloaking was introduced by Sir John Pendry (Imperial College, UK) and his collaborators in 2006 (Ref. 1).

The cloak of invisibility modeled here is a concentric spherical shell, whose interior surface represents the concealed cavity. Omnidirectional cloaks require anisotropic material properties, which can be calculated using the transformation optics theory (Ref. 1). Although ray and beam bending can be achieved with a gradient of isotropic refractive index, index gradient alone is not sufficient for omnidirectional invisibility. This can be shown by means of the uniqueness theorem that applies to scattering problems involving bodies composed of isotropic materials (Ref. 2).

The refractive index in the azimuthal directions (normal to the radial direction) experiences a gradual change from unity on the exterior surface of the cloak, where it matches free space, down to zero on the interior surface. With a proper choice of index distribution, you can ensure that any ray hitting the cloak never reaches the interior surface, and thus never probes the object. The refractive index in the radial direction is not continuous in this particular cloak design. The resulting index discontinuity at the exterior surface does not lead to reflections because only the index in the tangential direction affects reflectivity.

This model demonstrates the use of optical tracing for studying optically large gradient-index structures with anisotropic optical properties. Additionally, the model introduces a smoothing technique for handling discontinuities of refractive index on curved surfaces, which are typical in conventional optical devices such as lenses.

Model Definition

There is no explicit support for modeling geometrical optics in the Particle Tracing Module, but an analogy between the Hamilton equations and the equations for rays in the zero wavelength limit allows us to solve the problem. The analogy is as follows:

•

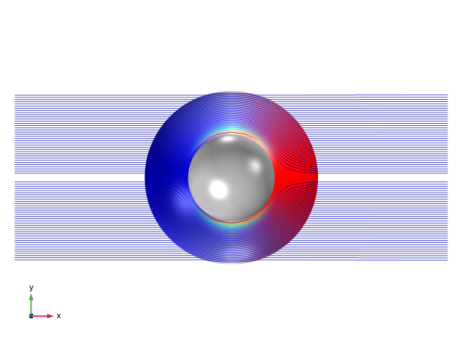
The wave vector, k (SI unit: 1/m) plays the same role in geometrical optics as the momentum, p, of particles in classical mechanics.

•

The angular frequency, ω (SI unit: 1/s) plays the role of the Hamiltonian, H.

For a classical particle, Hamilton’s equations are:

and using the analogy above:

•

The particle mass should be set to 1.

For geometrical optics, the angular frequency is given by

where n (dimensionless) is the refractive index of the material and c (SI unit: m/s) is the speed of light in a vacuum. For vacuum, the refractive index is simply 1. Inside the cloak, the refractive index is anisotropic, so it is more convenient to express the wave vector using spherical coordinates:

The angular frequency is hence given by:

Results and Discussion

The ray trajectories are plotted in Figure 1. The rays reach the cloak and bend around the inner sphere, which would appear invisible to an observer.

Figure 1: Plot of the light rays traveling through the cloak.

A better way of determining whether the incoming rays are returned to their original trajectory is by using a or plot. Figure 2 shows a in the yz-plane at the initial time step (red dots) and at the final time step (blue dots). In this Poincaré map, the horizontal position indicates the particle’s y-coordinate and the vertical position indicates the particle’s z-coordinate. The particle position after traveling through the cloak is almost exactly the same as it was initially.

Figure 3 shows the change in the particles’ position in the yz-plane after traveling through the cloaking device. The particles at the maximum and minimum y-coordinates have greater absolute error in their final positions despite being deflected at lower angles compared to the particles passing through the middle of the cloak. The higher absolute error may be due to these particles entering the anisotropic domain at a very oblique angle of incidence.

Figure 2: Poincaré map in the yz-plane at for Poincaré sections at x = −1 (red) and x = 1 (blue).

Figure 3: Change in the y-component of the particle position after traveling through the cloaking device.

References

1. J. Pendry, D. Schurig, and D.R. Smith, “Controlling Electromagnetic Fields,” Science, vol. 312, no. 5781, pp. 1780–1782, 2006.

2. A.I. Nachman, “Reconstruction from Boundary Measurements,” Ann. Math., vol. 128, pp. 531–576, 1988.

Particle_Tracing_Module/Tutorials/ideal_cloak

Modeling Instructions

This model comes courtesy of Yaroslav Urzhumov, Center for Metamaterials and Integrated Plasmonics, Duke University.

From the menu, choose .

New

In the window, click

Model Wizard

In the window, click

In the tree, select .

Click .

Click

In the tree, select .

Click

Global Definitions

Specify the dimensions of the air domain and the cloak.

Parameters 1

In the window, under click .

In the window for , locate the section.

In the table, enter the following settings:


Name	Expression	Value	Description
L	1[m]	1 m	Box length
a	0.2[m]	0.2 m	Inner radius
b	0.4[m]	0.4 m	Outer radius
n_air	1	1	Refractive index of air
hmax	L*0.2	0.2 m	Maximum element size in volume
hmax_cloak	b*0.05	0.02 m	Maximum element size in cloak

Geometry 1

Block 1 (blk1)

In the toolbar, click

In the window for , locate the section.

In the text field, type 2*L.

Locate the section. From the list, choose .

Sphere 1 (sph1)

In the toolbar, click

In the window for , locate the section.

In the text field, type a.

Sphere 2 (sph2)

In the toolbar, click

In the window for , locate the section.

In the text field, type b.

Click

Click the

button in the toolbar.

Definitions

Now add the expressions which transform the refractive index of the cloak from Cartesian to spherical coordinates. The wave vector must also be transformed.

Variables 1

In the toolbar, click

and choose .

Load the variable definitions from a file.

In the window for , locate the section.

Click

Browse to the model’s Application Libraries folder and double-click the file ideal_cloak_variables.txt.

Mathematical Particle Tracing (pt)

Using the analogy presented in the introduction section above, enter an expression for the angular frequency, which is called H_photon in this case.

In the window, under click .

In the window for , locate the section.

From the list, choose .

Particle Properties 1

In the window, under click .

In the window for , locate the section.

In the H text field, type H_photon.

Locate the section. In the mp text field, type 1.

Release from Grid 1

Next, release the particles in the y direction for fixed x- and z-coordinates.

In the toolbar, click

and choose .

In the window for , locate the section.

In the qx,0 text field, type -1.

In the qy,0 text field, type range(-0.38,0.01,-0.02) range(0.02,0.01,0.38).

Locate the section. Specify the v0 vector as


1	x
0	y
0	z

Because the Hamiltonian formulation is being used to model rays, the settings entered for the determine the initial wave vector direction, not the velocity of the model particles.

Mesh 1

The mesh needs to be fine in the cloak region so that the particle trajectories can be computed to a high degree of accuracy.

Free Tetrahedral 1

In the toolbar, click

Size 1

Right-click and choose .

In the window for , locate the section.

From the list, choose .

Select Domain 2 only.

Click to expand the section. Locate the section. Click the button.

Locate the section. Select the check box.

In the associated text field, type hmax_cloak.

Select the check box.

In the associated text field, type hmax_cloak/2.

Size

In the window, click .

In the window for , locate the section.

Click the button.

Locate the section. In the text field, type hmax.

In the text field, type hmax/2.

In the window, right-click and choose .

Study 1

To accurately compute the particle trajectories in an anisotropic medium, the default solver tolerances need to be made more strict. Do this by first showing the default solver, and then reducing the relative and absolute tolerances.

Step 1: Time Dependent

In the window, under click .

In the window for , locate the section.

Click

In the dialog box, type 2[m]/c_const in the text field.

From the list, choose .

In the text field, type 301.

Click .

In the window for , locate the section.

From the list, choose .

In the text field, type 1e-6.

Solution 1 (sol1)

In the toolbar, click

In the window, expand the node.

In the window, click .

In the window for , click to expand the section.

From the list, choose .

In the window, click .

In the window for , click to expand the section.

In the toolbar, click

Results

Particle Trajectories (pt)

The path of the rays is best visualized by adding selections for the cloak inner and outer surfaces.

In the window for , locate the section.

Clear the check box.

Click to expand the section. From the list, choose .

In the window, expand the node.

Particle Trajectories 1

In the window, expand the node, then click .

In the window for , locate the section.

Find the subsection. From the list, choose .

Color Expression 1

In the window, click .

In the window for , locate the section.

Clear the check box.

Surface 1

In the window, right-click and choose .

In the window for , locate the section.

From the list, choose .

Locate the section. In the text field, type cos_phi.

Locate the section. From the list, choose .

Clear the check box.

Selection 1

Right-click and choose .

Select Boundaries 6, 8, 14, and 18 only.

Surface 2

In the window, right-click and choose .

In the window for , locate the section.

From the list, choose .

Locate the section. From the list, choose .

From the list, choose .

Selection 1

Right-click and choose .

Select Boundaries 10–13, 16, 17, 19, and 20 only.

Definitions

In the window, under click .

In the window for , locate the section.

Clear the check box.

Results

Particle Trajectories 1

In the window, under click .

In the toolbar, click

Click the

button in the toolbar. The plot should look like Figure 1.

To see how well the cloak performs, look at the rays in phase space before and after they pass through the cloak. You can do this in two different ways.

The first method is to define a pair of datasets on the incoming and outgoing sides of the cloak, then plot a of the ray positions as they intersect each plane.

Cut Plane 1

In the window, expand the node.

Right-click and choose .

In the window for , locate the section.

From the list, choose .

Locate the section. In the text field, type -0.99.

Cut Plane 2

Right-click and choose .

In the window for , locate the section.

In the text field, type 0.99.

Ray Position Relative to Initial Position

In the toolbar, click

In the window for , type Ray Position Relative to Initial Position in the text field.

Locate the section. Select the check box.

In the associated text field, type y-position (m).

Select the check box.

In the associated text field, type z-position (m).

Poincaré Map 1

In the toolbar, click

and choose .

In the window for , locate the section.

From the list, choose .

In the toolbar, click

Click the

button in the toolbar.

Poincaré Map 2

Right-click and choose .

In the window for , locate the section.

From the list, choose .

Locate the section. From the list, choose .

Select the check box.

In the associated text field, type 0.7.

Click to expand the section. From the list, choose .

In the toolbar, click

Click the

button in the toolbar. The plot should look like Figure 2.

The second method is to construct a of the rays and verify that their positions and velocities are the same before and after passing through the cloak.

Change in Lateral Position

In the toolbar, click