PDF
Rotors Connected Through Helical Gears
Introduction
This example illustrates the modeling of multiple rotors connected through helical gears. The presence of gears in the system induces lateral as well as torsional vibration in the rotors. An eigenfrequency analysis is performed for different speeds of the driver shaft in order to compute the critical speeds. The frequency response of the system is also computed for a range of frequencies, assuming the speed of the driver shaft to be the same as the excitation frequency. A transient analysis is performed for the given speed and load to compute the orbit and the dynamic transmission error of the gears as well as the forces acting on the bearings.
Model Definition
The model consists of three shafts connected through two pairs of helical gears. The helical gear on the first (driver) shaft transfers rotation to the larger helical gear of the second (intermediate) shaft. The smaller helical gear of the second (intermediate) shaft transfers rotation to the helical gear of the third (driven) shaft. All three shafts are simply supported at both the ends using journal bearings.
The geared rotor geometry is shown in Figure 1 below.
Figure 1: Geared rotor geometry.
Shafts
All the shafts are of the same dimensions and made of structural steel. The shafts’ diameter and length are 40 mm and 600 mm, respectively.
In the time-dependent analysis, the angular speed of the driver shaft is assumed to be 1500 rpm. In the eigenfrequency, it is varied from 0 to 9000 rpm whereas in the frequency domain analysis, it is assumed to be the same as the excitation frequency. The angular speed of the other two shafts is computed using the gear ratio.
Bearings
Each shaft is supported by journal bearings at both the ends. All the bearings are assumed to have constant isotropic stiffness with a value of 107 N/m.
Gears
The properties of the helical gears are given in the Table 1.
Table 1: gear properties.
Property
value
Number of teeth (Gear-1)
20
Pitch diameter (Gear-1)
100 mm
Number of teeth (Gear-2)
30
Pitch diameter (Gear-2)
150 mm
Pressure angle
25º
Helix angle
30º
Gear ratio
1.5
Gear mesh stiffness
2·106 N/m
The density of the gears, used to compute the inertial properties, is same as the shaft density.
constraints and loads
•
The axial rotation is constrained at one end of the driver shaft.
•
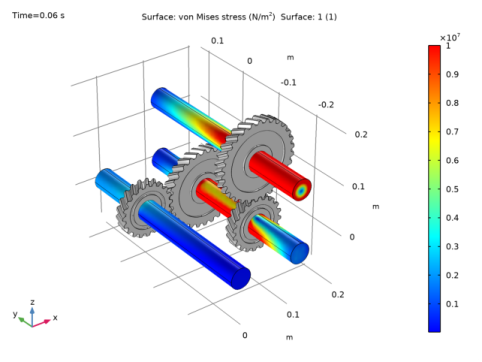
In the time dependent analysis, a resisting load torque of 102 Nm is applied at the opposite end of the driven shaft.
Critical Speeds of a Rotor
The angular speeds of a rotor that match its natural frequencies are known as the critical speeds. Approximate values of the critical speeds of a rotor can be obtained as the rotor’s eigenfrequencies computed while neglecting rotating frame forces. However, to predict the eigenfrequencies accurately, the rotating frame forces for a range of angular speeds must be included in the computations. The Campbell diagram, showing the variation of eigenfrequencies with angular speed, can be drawn and accurate critical speeds can be computed. A Campbell plot using the Solid Rotor interface plots the frequencies in the rotating frame. In such a plot critical speeds are obtained by the intersection of the backward whirl frequency curve with ω = 2Ω curve and intersection of the forward whirl curve with the x-axis. In the rotating frame, generally the backward whirl frequencies increase with the rotor speed and forward whirl frequencies decrease.
Results and Discussion
There are five points where the ω = 2Ω curve intersects with the eigenfrequency curve. These are the critical speeds of the rotor system below 9000 rpm and they are listed in the Table 2.
Table 2: critical speeds of the rotor.
Mode
Critical speeds (rad/s)
First
281.76
Second
473.68
Third
548.91
Fourth
595.05
Fifth
668.68
.
Figure 2: The second mode of the geared rotors at 9000 rpm.
Figure 2 shows the second mode (f = 113.4 Hz) of the geared rotors at 9000 rpm. The Campbell Plot, showing the variation of eigenfrequencies with the rotational speed, is shown in Figure 3. The three critical speeds of the rotor, where the straight line (ω = 2Ω) intersects the eigenfrequency curves, can be seen.
Figure 3: Campbell diagram.
The frequency response curves for the gear displacement and rotation are shown in Figure 4 and Figure 5. It can be seen that both the displacement and rotation of all gears have a peak around the critical speeds computed from the Campbell plot.
Figure 4: Frequency response of the x-direction displacement of all the gears.
Figure 5: Frequency response of the z-direction rotation of all the gears.
Figure 6 shows the von Mises stress distribution in the shafts. It can be observed that the twisting of different shafts start from the end of the driven shaft, where the load is applied, to the opposite end of the driver shaft, where the axial rotation is constrained. The remaining parts of the shafts experience mostly the bending load generated due to gearing action.
Figure 6: von Mises stress distribution in the shafts at a particular instance.
Figure 7 and Figure 8 show the orbits of the gear centers in the rotating and the fixed frame of reference, respectively. It can be observed that in the rotating frame of reference, all the gears are settled in different directions except gear-2 and gear-3, as they are both mounted on the intermediate shaft.
The gear mesh is assumed to be elastic, which causes an error while transferring rotation from one shaft to another. This error, known as the dynamic transmission error (DTE), is shown in Figure 9.
Finally, Figure 10 shows the time variation of the bearing loads on one of the journal bearings.
Figure 7: Orbits of gear center in the rotating frame of reference.
Figure 8: Orbits of gear center in the fixed frame of reference.
Figure 9: Time variation of the dynamic transmission error (DTE) of both gear pairs.
Figure 10: Time variation of the bearing forces.
Notes About the COMSOL Implementation
The Solid Rotor physics interface is used to model multiple rotors connected through gears.
To model multiple rotors, use Rotor Axis and Change Rotor Speed features to define the axis of rotation and speed of each rotor. In cases where two shafts are connected through gears, the shaft speeds are related through the gear ratio.
The gear mesh stiffness is assumed constant. However, in general it is a function of gear rotation. The varying gear mesh stiffness causes additional sustained vibration in the system.
It is possible to visualize results in both the rotating as well as the fixed frame of reference. In this model, the fixed frame of reference is used when animating the results.
Application Library path: Multibody_Dynamics_Module/Tutorials,_Transmission/geared_rotors
Modeling Instructions
From the File menu, choose New.
New
In the New window, click  Model Wizard.
Model Wizard
1
In the Model Wizard window, click  3D.
2
In the Select Physics tree, select Structural Mechanics>Rotordynamics>Solid Rotor (rotsld).
3
Click Add.
4
Click  Study.
5
In the Select Study tree, select General Studies>Eigenfrequency.
6
Click  Done.
Global Definitions
Parameters 1
1
In the Model Builder window, under Global Definitions click Parameters 1.
2
In the Settings window for Parameters, locate the Parameters section.
3
Click  Load from File.
4
Browse to the model’s Application Libraries folder and double-click the file geared_rotors_parameters.txt.
Definitions
Step 1 (step1)
1
In the Model Builder window, expand the Component 1 (comp1)>Definitions node.
2
Right-click Definitions and choose Functions>Step.
3
In the Settings window for Step, locate the Parameters section.
4
In the Location text field, type t_end/12.
5
Click to expand the Smoothing section. In the Size of transition zone text field, type t_end/6.
Geometry 1
Import 1 (imp1)
1
In the Home toolbar, click  Import.
2
In the Settings window for Import, locate the Import section.
3
Click Browse.
4
Browse to the model’s Application Libraries folder and double-click the file geared_rotors.mphbin.
5
Click Import.
Form Union (fin)
1
In the Model Builder window, under Component 1 (comp1)>Geometry 1 click Form Union (fin).
2
In the Settings window for Form Union/Assembly, locate the Form Union/Assembly section.
3
From the Action list, choose Form an assembly.
4
Clear the Create pairs check box.
5
In the Home toolbar, click  Build All.
Add Material
1
In the Home toolbar, click  Add Material to open the Add Material window.
2
Go to the Add Material window.
3
In the tree, select Built-in>Structural steel.
4
Click Add to Component in the window toolbar.
5
In the Home toolbar, click  Add Material to close the Add Material window.
Solid Rotor (rotsld)
This model has multiple rotors having different axis of rotation as well as different speeds. So first set the axis of rotation and speed for all three rotors.
1
In the Model Builder window, under Component 1 (comp1) click Solid Rotor (rotsld).
2
In the Settings window for Solid Rotor, locate the Rotor Speed section.
3
In the text field, type omega.
First Support 1
1
In the Model Builder window, expand the Rotor Axis 1 node, then click First Support 1.
2
In the Settings window for First Support, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 97-98 in the Selection text field.
5
Click OK.
Second Support 1
1
In the Model Builder window, click Second Support 1.
2
In the Settings window for Second Support, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 119-120 in the Selection text field.
5
Click OK.
Rotor Axis 2
1
In the Physics toolbar, click  Domains and choose Rotor Axis.
2
Select Domains 5–7 only.
First Support 1
1
In the Model Builder window, expand the Rotor Axis 2 node, then click First Support 1.
2
In the Settings window for First Support, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 744, 756 in the Selection text field.
5
Click OK.
Second Support 1
1
In the Model Builder window, click Second Support 1.
2
In the Settings window for Second Support, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 749, 761 in the Selection text field.
5
Click OK.
Rotor Axis 3
1
In the Physics toolbar, click  Domains and choose Rotor Axis.
2
Select Domains 3 and 4 only.
First Support 1
1
In the Model Builder window, expand the Rotor Axis 3 node, then click First Support 1.
2
In the Settings window for First Support, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 355, 363 in the Selection text field.
5
Click OK.
Second Support 1
1
In the Model Builder window, click Second Support 1.
2
In the Settings window for Second Support, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 358, 366 in the Selection text field.
5
Click OK.
Change Rotor Speed 1
1
In the Physics toolbar, click  Domains and choose Change Rotor Speed.
2
Select Domains 5–7 only.
3
In the Settings window for Change Rotor Speed, locate the Rotor Speed section.
4
In the text field, type -omega/gr.
Change Rotor Speed 2
1
In the Physics toolbar, click  Domains and choose Change Rotor Speed.
2
Select Domains 3 and 4 only.
3
In the Settings window for Change Rotor Speed, locate the Rotor Speed section.
4
In the text field, type omega/gr^2.
Fixed Axial Rotation 1
1
In the Model Builder window, click Fixed Axial Rotation 1.
2
Select Boundary 57 only.
Now define helical gears to connect the three rotors.
Helical Gear 1
1
In the Physics toolbar, click  Domains and choose Helical Gear.
2
Select Domain 1 only.
3
In the Settings window for Helical Gear, locate the Gear Properties section.
4
In the n text field, type n1.
5
In the dp text field, type d1.
6
In the α text field, type alpha.
7
In the β text field, type beta.
8
Locate the Center of Rotation section. From the list, choose Centroid of selected entities.
9
From the Entity level list, choose Point.
Center of Rotation: Point 1
1
In the Model Builder window, expand the Helical Gear 1 node, then click Center of Rotation: Point 1.
2
In the Settings window for Center of Rotation: Point, locate the Point Selection section.
3
Click  Paste Selection.
4
In the Paste Selection dialog box, type 101, 115 in the Selection text field.
5
Click OK.
Helical Gear 2
1
In the Model Builder window, under Component 1 (comp1)>Solid Rotor (rotsld) right-click Helical Gear 1 and choose Duplicate.
2
In the Settings window for Helical Gear, locate the Domain Selection section.
3
Click  Clear Selection.
4
Select Domain 5 only.
5
Locate the Gear Properties section. In the n text field, type n2.
6
In the dp text field, type d2.
7
In the β text field, type -beta.
Center of Rotation: Point 1
1
In the Model Builder window, expand the Helical Gear 2 node, then click Center of Rotation: Point 1.
2
In the Settings window for Center of Rotation: Point, locate the Point Selection section.
3
Click  Clear Selection.
4
Click  Paste Selection.
5
In the Paste Selection dialog box, type 747, 760 in the Selection text field.
6
Click OK.
Helical Gear 3
1
In the Model Builder window, under Component 1 (comp1)>Solid Rotor (rotsld) right-click Helical Gear 1 and choose Duplicate.
2
In the Settings window for Helical Gear, locate the Domain Selection section.
3
Click  Clear Selection.
4
Select Domain 6 only.
Center of Rotation: Point 1
1
In the Model Builder window, expand the Helical Gear 3 node, then click Center of Rotation: Point 1.
2
In the Settings window for Center of Rotation: Point, locate the Point Selection section.
3
Click  Clear Selection.
4
Click  Paste Selection.
5
In the Paste Selection dialog box, type 745, 758 in the Selection text field.
6
Click OK.
Helical Gear 4
1
In the Model Builder window, under Component 1 (comp1)>Solid Rotor (rotsld) right-click Helical Gear 2 and choose Duplicate.
2
In the Settings window for Helical Gear, locate the Domain Selection section.
3
Click  Clear Selection.
4
Select Domain 3 only.
Center of Rotation: Point 1
1
In the Model Builder window, expand the Helical Gear 4 node, then click Center of Rotation: Point 1.
2
In the Settings window for Center of Rotation: Point, locate the Point Selection section.
3
Click  Clear Selection.
4
Click  Paste Selection.
5
In the Paste Selection dialog box, type 357, 364 in the Selection text field.
6
Click OK.
Gear Pair 1
1
In the Physics toolbar, click  Global and choose Gear Pair.
2
In the Settings window for Gear Pair, locate the Gear Selection section.
3
From the Wheel list, choose Helical Gear 1.
4
From the Pinion list, choose Helical Gear 2.
5
Locate the Gear Pair Properties section. Select the Include gear elasticity check box.
Gear Elasticity 1
1
In the Model Builder window, expand the Gear Pair 1 node, then click Gear Elasticity 1.
2
In the Settings window for Gear Elasticity, locate the Mesh Stiffness section.
3
From the Specify list, choose Total stiffness of gear pair.
4
In the kg text field, type kg.
Gear Pair 2
1
In the Physics toolbar, click  Global and choose Gear Pair.
2
In the Settings window for Gear Pair, locate the Gear Selection section.
3
From the Wheel list, choose Helical Gear 3.
4
From the Pinion list, choose Helical Gear 4.
5
Locate the Gear Pair Properties section. Select the Include gear elasticity check box.
Gear Elasticity 1
1
In the Model Builder window, expand the Gear Pair 2 node, then click Gear Elasticity 1.
2
In the Settings window for Gear Elasticity, locate the Mesh Stiffness section.
3
From the Specify list, choose Total stiffness of gear pair.
4
In the kg text field, type kg.
Now define journal bearings to provide the support for the rotors on both ends.
Journal Bearing 1
1
In the Physics toolbar, click  Boundaries and choose Journal Bearing.
2
Select Boundary 52 only.
3
In the Settings window for Journal Bearing, locate the Bearing Orientation section.
4
Specify the Orientation vector defining local y direction vector as
1
x
0
y
0
z
5
Locate the Bearing Properties section. From the Bearing model list, choose Total spring and damping constant.
6
In the ku table, enter the following settings:
kb
0
0
kb
Journal Bearing 2
1
Right-click Journal Bearing 1 and choose Duplicate.
2
In the Settings window for Journal Bearing, locate the Boundary Selection section.
3
Click  Clear Selection.
4
Select Boundary 57 only.
5
Locate the Bearing Properties section. In the ku table, enter the following settings:
kb
0
0
kb
6
Clear the Constrain axial motion check box.
Journal Bearing 3
1
Right-click Journal Bearing 2 and choose Duplicate.
2
In the Settings window for Journal Bearing, locate the Boundary Selection section.
3
Click  Clear Selection.
4
Select Boundary 397 only.
5
Locate the Bearing Properties section. In the ku table, enter the following settings:
kb
0
0
kb
6
Select the Constrain axial motion check box.
Journal Bearing 4
1
Right-click Journal Bearing 3 and choose Duplicate.
2
In the Settings window for Journal Bearing, locate the Boundary Selection section.
3
Click  Clear Selection.
4
Select Boundary 406 only.
5
Locate the Bearing Properties section. In the ku table, enter the following settings:
kb
0
0
kb
6
Clear the Constrain axial motion check box.
Journal Bearing 5
1
Right-click Journal Bearing 4 and choose Duplicate.
2
In the Settings window for Journal Bearing, locate the Boundary Selection section.
3
Click  Clear Selection.
4
Select Boundary 191 only.
5
Locate the Bearing Properties section. In the ku table, enter the following settings:
kb
0
0
kb
Journal Bearing 6
1
Right-click Journal Bearing 5 and choose Duplicate.
2
In the Settings window for Journal Bearing, locate the Boundary Selection section.
3
Click  Clear Selection.
4
Select Boundary 196 only.
5
Locate the Bearing Properties section. In the ku table, enter the following settings:
kb
0
0
kb
6
Select the Constrain axial motion check box.
Applied Torque 1
1
In the Physics toolbar, click  Boundaries and choose Applied Torque.
2
Select Boundary 191 only.
3
In the Settings window for Applied Torque, locate the Torque section.
4
In the Tax text field, type Text*step1(t[1/s]).
Mesh 1
1
In the Model Builder window, under Component 1 (comp1) click Mesh 1.
2
In the Settings window for Mesh, locate the Physics-Controlled Mesh section.
3
From the Element size list, choose Coarse.
4
Click  Build All.
Study 1: Eigenfrequency
1
In the Model Builder window, click Study 1.
2
In the Settings window for Study, type Study 1: Eigenfrequency in the Label text field.
Parametric Sweep
1
In the Study toolbar, click  Parametric Sweep.
2
In the Settings window for Parametric Sweep, locate the Study Settings section.
3
Click  Add.
4
In the table, enter the following settings:
Parameter name
Parameter value list
Parameter unit
omega (Angular speed of driver shaft)
range(0,500,9000)
rpm
Step 1: Eigenfrequency
1
In the Model Builder window, click Step 1: Eigenfrequency.
2
In the Settings window for Eigenfrequency, locate the Study Settings section.
3
Select the Desired number of eigenfrequencies check box.
4
In the associated text field, type 10.
5
In the Study toolbar, click  Compute.
Results
Mode Shape (rotsld)
Use the following instructions to plot the mode shape and Campbell diagram as shown in Figure 2 and Figure 3 respectively.
1
In the Settings window for 3D Plot Group, locate the Data section.
2
From the Eigenfrequency (Hz) list, choose 113.38.
3
In the Mode Shape (rotsld) toolbar, click  Plot.
4
Click the  Zoom Extents button in the Graphics toolbar.
Backward Whirl Mode
Default Campbell Plot, Rotating Frame segregates the whirl modes, namely, forward and backward. Because of the multiple rotors rotating at different speeds whirl modes in all the rotors will not be identical. Therefore, automatic identification of the whirl mode is not correct in this case. Delete the Backward Whirl Mode node in this plot group.
1
In the Model Builder window, expand the Campbell Plot, Rotating Frame (rotsld) node.
2
Right-click Backward Whirl Mode and choose Delete.
Campbell Plot, Rotating Frame (rotsld)
1
In the Model Builder window, click Campbell Plot, Rotating Frame (rotsld).
2
In the Campbell Plot, Rotating Frame (rotsld) toolbar, click  Plot.
3
Click the  Zoom Extents button in the Graphics toolbar.
Campbell Plot, Fixed Frame (rotsld)
Delete the default Campbell Plot, Fixed Frame because the rotating frame to fixed frame transformation in the present case is not unique.
1
In the Model Builder window, right-click Campbell Plot, Fixed Frame (rotsld) and choose Delete.
Add Study
1
In the Home toolbar, click  Add Study to open the Add Study window.
2
Go to the Add Study window.
3
Find the Studies subsection. In the Select Study tree, select General Studies>Frequency Domain.
4
Click Add Study in the window toolbar.
5
In the Home toolbar, click  Add Study to close the Add Study window.
Study 2: Frequency Domain
1
In the Model Builder window, click Study 2.
2
In the Settings window for Study, type Study 2: Frequency Domain in the Label text field.
3
Locate the Study Settings section. Clear the Generate default plots check box.
Parametric Sweep
1
In the Study toolbar, click  Parametric Sweep.
2
In the Settings window for Parametric Sweep, locate the Study Settings section.
3
Click  Add.
4
In the table, enter the following settings:
Parameter name
Parameter value list
Parameter unit
omega (Angular speed of driver shaft)
range(1000,1000,2000) range(2500,50,6500) range(7000,1000,9000)
rpm
Step 1: Frequency Domain
1
In the Model Builder window, click Step 1: Frequency Domain.
2
In the Settings window for Frequency Domain, locate the Study Settings section.
3
In the Frequencies text field, type 2*omega.
4
Locate the Physics and Variables Selection section. Select the Modify model configuration for study step check box.
5
In the Physics and variables selection tree, select Component 1 (comp1)>Solid Rotor (rotsld)>Applied Torque 1.
6
Click  Disable.
Set the Null-space function to Orthonormal for better constraint handling.
Solution 22 (sol22)
1
In the Study toolbar, click  Show Default Solver.
2
In the Model Builder window, expand the Solution 22 (sol22) node.
3
In the Model Builder window, expand the Study 2: Frequency Domain>Solver Configurations>Solution 22 (sol22)>Stationary Solver 1 node, then click Advanced.
4
In the Settings window for Advanced, locate the General section.
5
From the Null-space function list, choose Orthonormal.
6
In the Study toolbar, click  Compute.
Results
Use the following instructions to plot the frequency response curves as shown in Figure 4 and Figure 5 respectively.
1D Plot Group 4
1
In the Home toolbar, click  Add Plot Group and choose 1D Plot Group.
2
In the Settings window for 1D Plot Group, locate the Data section.
3
From the Dataset list, choose Study 2: Frequency Domain/Parametric Solutions 2 (sol23).
Global 1
1
Right-click 1D Plot Group 4 and choose Global.
2
In the Settings window for Global, locate the y-Axis Data section.
3
In the table, enter the following settings:
Expression
Unit
Description
abs(rotsld.hlg1.u)
m
 
abs(rotsld.hlg2.u)
m
 
abs(rotsld.hlg3.u)
m
 
abs(rotsld.hlg4.u)
m
 
4
Locate the x-Axis Data section. From the Axis source data list, choose Outer solutions.
5
From the Parameter list, choose Expression.
6
In the Expression text field, type 2*pi[rad]*omega.
7
Select the Description check box.
8
In the associated text field, type omega.
9
Click to expand the Coloring and Style section. Find the Line style subsection. From the Line list, choose Cycle.
10
In the Width text field, type 3.
11
Click to expand the Legends section. From the Legends list, choose Manual.
12
In the table, enter the following settings:
Legends
Gear-1
Gear-2
Gear-3
Gear-4
Frequency response: x-displacement
1
In the Model Builder window, under Results click 1D Plot Group 4.
2
In the Settings window for 1D Plot Group, type Frequency response: x-displacement in the Label text field.
3
Click to expand the Title section. From the Title type list, choose Label.
4
Locate the Plot Settings section. Select the y-axis label check box.
5
In the associated text field, type x-displacement (m).
6
Locate the Axis section. Select the y-axis log scale check box.
7
In the Frequency response: x-displacement toolbar, click  Plot.
8
Click the  Zoom Extents button in the Graphics toolbar.
Frequency response: x-displacement 1
Right-click Frequency response: x-displacement and choose Duplicate.
Global 1
1
In the Model Builder window, expand the Frequency response: x-displacement 1 node, then click Global 1.
2
In the Settings window for Global, locate the y-Axis Data section.
3
In the table, enter the following settings:
Expression
Unit
Description
abs(rotsld.hlg1.thz)
rad
 
abs(rotsld.hlg2.thz)
rad
 
abs(rotsld.hlg3.thz)
rad
 
abs(rotsld.hlg4.thz)
rad
 
Frequency response: z-rotation
1
In the Model Builder window, under Results click Frequency response: x-displacement 1.
2
In the Settings window for 1D Plot Group, type Frequency response: z-rotation in the Label text field.
3
Locate the Plot Settings section. In the y-axis label text field, type z-rotation (rad).
4
In the Frequency response: z-rotation toolbar, click  Plot.
5
Click the  Zoom Extents button in the Graphics toolbar.
Add Study
1
In the Home toolbar, click  Add Study to open the Add Study window.
2
Go to the Add Study window.
3
Find the Studies subsection. In the Select Study tree, select General Studies>Time Dependent.
4
Click Add Study in the window toolbar.
5
In the Home toolbar, click  Add Study to close the Add Study window.
Study 3: Time Dependent
1
In the Model Builder window, click Study 3.
2
In the Settings window for Study, type Study 3: Time Dependent in the Label text field.
Step 1: Time Dependent
1
In the Model Builder window, under Study 3: Time Dependent click Step 1: Time Dependent.
2
In the Settings window for Time Dependent, locate the Study Settings section.
3
In the Output times text field, type range(0,t_end/150,t_end).
4
In the Home toolbar, click  Compute.
Results
Study 3: Time Dependent/Solution 110 (sol110)
Use the following instructions to plot the von-Mises stress and gear orbits as shown in Figure 6, Figure 7, and Figure 8 respectively.
Study 3: Time Dependent/Solution 110 (6) (sol110)
1
In the Model Builder window, expand the Results>Datasets node.
2
Right-click Results>Datasets>Study 3: Time Dependent/Solution 110 (sol110) and choose Duplicate.
Selection
1
In the Results toolbar, click  Attributes and choose Selection.
2
In the Settings window for Selection, locate the Geometric Entity Selection section.
3
From the Geometric entity level list, choose Domain.
4
Select Domains 1, 3, 5, and 6 only.
Surface
In the Model Builder window, expand the Results>Stress (rotsld) node.
Deformation
1
In the Model Builder window, expand the Surface node, then click Deformation.
2
In the Settings window for Deformation, click Replace Expression in the upper-right corner of the Expression section. From the menu, choose Component 1 (comp1)>Solid Rotor>Displacement>rotsld.us_fix,...,rotsld.ws_fix - Displacement field, fixed frame.
3
Locate the Scale section. Select the Scale factor check box.
4
In the associated text field, type 1.
Surface 2
1
In the Model Builder window, right-click Surface and choose Duplicate.
2
In the Settings window for Surface, locate the Data section.
3
From the Dataset list, choose Study 3: Time Dependent/Solution 110 (6) (sol110).
4
Locate the Expression section. In the Expression text field, type 1.
5
Locate the Coloring and Style section. From the Coloring list, choose Uniform.
6
From the Color list, choose Gray.
Surface
1
In the Model Builder window, click Surface.
2
In the Settings window for Surface, click to expand the Range section.
3
Select the Manual color range check box.
4
In the Maximum text field, type 1e7.
5
In the Stress (rotsld) toolbar, click  Plot.
6
Click the  Zoom Extents button in the Graphics toolbar.
Cut Point 3D 1
1
In the Results toolbar, click  Cut Point 3D.
2
In the Settings window for Cut Point 3D, locate the Data section.
3
From the Dataset list, choose Study 3: Time Dependent/Solution 110 (5) (sol110).
4
Locate the Point Data section. In the X text field, type rotsld.hlg1.xcx, rotsld.hlg2.xcx, rotsld.hlg3.xcx, rotsld.hlg4.xcx.
5
In the Y text field, type rotsld.hlg1.xcy, rotsld.hlg2.xcy, rotsld.hlg3.xcy, rotsld.hlg4.xcy.
6
In the Z text field, type rotsld.hlg1.xcz, rotsld.hlg2.xcz, rotsld.hlg3.xcz, rotsld.hlg4.xcz.
1D Plot Group 9
1
In the Results toolbar, click  1D Plot Group.
2
In the Settings window for 1D Plot Group, locate the Data section.
3
From the Dataset list, choose Cut Point 3D 1.
Point Graph 1
1
Right-click 1D Plot Group 9 and choose Point Graph.
2
In the Settings window for Point Graph, locate the y-Axis Data section.
3
In the Expression text field, type w.
4
Locate the x-Axis Data section. From the Parameter list, choose Expression.
5
In the Expression text field, type u.
6
Click to expand the Coloring and Style section. Find the Line style subsection. From the Line list, choose Cycle.
7
In the Width text field, type 3.
8
Click to expand the Legends section. Select the Show legends check box.
9
From the Legends list, choose Manual.
10
In the table, enter the following settings:
Legends
Gear-1
Gear-2
Gear-3
Gear-4
Gear orbits, rotating frame
1
In the Model Builder window, under Results click 1D Plot Group 9.
2
In the Settings window for 1D Plot Group, type Gear orbits, rotating frame in the Label text field.
3
Locate the Title section. From the Title type list, choose Label.
4
Locate the Axis section. Select the Preserve aspect ratio check box.
5
Locate the Legend section. From the Position list, choose Lower left.
6
In the Gear orbits, rotating frame toolbar, click  Plot.
7
Click the  Zoom Extents button in the Graphics toolbar.
Gear orbits, rotating frame 1
Right-click Gear orbits, rotating frame and choose Duplicate.
Point Graph 1
1
In the Model Builder window, expand the Gear orbits, rotating frame 1 node, then click Point Graph 1.
2
In the Settings window for Point Graph, locate the y-Axis Data section.
3
In the Expression text field, type rotsld.ws_fix.
4
Locate the x-Axis Data section. In the Expression text field, type rotsld.us_fix.
Gear orbits, fixed frame
1
In the Model Builder window, under Results click Gear orbits, rotating frame 1.
2
In the Settings window for 1D Plot Group, type Gear orbits, fixed frame in the Label text field.
3
In the Gear orbits, fixed frame toolbar, click  Plot.
4
Click the  Zoom Extents button in the Graphics toolbar.
1D Plot Group 11
Use the following instructions to plot the gear transmission errors and bearing forces as shown in Figure 9 and Figure 10 respectively.
1
In the Home toolbar, click  Add Plot Group and choose 1D Plot Group.
2
In the Settings window for 1D Plot Group, locate the Data section.
3
From the Dataset list, choose Study 3: Time Dependent/Solution 110 (5) (sol110).
Global 1
1
Right-click 1D Plot Group 11 and choose Global.
2
In the Settings window for Global, locate the y-Axis Data section.
3
In the table, enter the following settings:
Expression
Unit
Description
rotsld.grp1.th_el
deg
Transmission error (elasticity)
rotsld.grp2.th_el
deg
Transmission error (elasticity)
4
Locate the Coloring and Style section. Find the Line style subsection. From the Line list, choose Cycle.
5
In the Width text field, type 3.
6
Locate the Legends section. From the Legends list, choose Manual.
7
In the table, enter the following settings:
Legends
Gear-1
Gear-2
Gear DTEs
1
In the Model Builder window, under Results click 1D Plot Group 11.
2
In the Settings window for 1D Plot Group, type Gear DTEs in the Label text field.
3
Locate the Title section. From the Title type list, choose Manual.
4
In the Title text area, type Dynamic Transmission Errors.
5
Locate the Legend section. From the Position list, choose Lower left.
6
In the Gear DTEs toolbar, click  Plot.
7
Click the  Zoom Extents button in the Graphics toolbar.
Cut Point 3D 2
1
In the Results toolbar, click  Cut Point 3D.
2
In the Settings window for Cut Point 3D, locate the Data section.
3
From the Dataset list, choose Study 3: Time Dependent/Solution 110 (5) (sol110).
4
Locate the Point Data section. In the X text field, type 0.
5
In the Y text field, type 1.25*d1.
6
In the Z text field, type 0.
7
Select the Snap to closest boundary check box.
1D Plot Group 12
1
In the Results toolbar, click  1D Plot Group.
2
In the Settings window for 1D Plot Group, locate the Data section.
3
From the Dataset list, choose Cut Point 3D 2.
Point Graph 1
1
Right-click 1D Plot Group 12 and choose Point Graph.
2
In the Settings window for Point Graph, locate the y-Axis Data section.
3
In the Expression text field, type rotsld.jrb2.f2.
4
Locate the Coloring and Style section. Find the Line style subsection. From the Line list, choose Cycle.
5
In the Width text field, type 3.
6
Locate the Legends section. From the Legends list, choose Manual.
7
Select the Show legends check box.
8
In the table, enter the following settings:
Legends
x-component
Point Graph 2
1
Right-click Point Graph 1 and choose Duplicate.
2
In the Settings window for Point Graph, locate the y-Axis Data section.
3
In the Expression text field, type rotsld.jrb2.f3.
4
Locate the Legends section. In the table, enter the following settings:
Legends
z-component
Bearing forces
1
In the Model Builder window, under Results click 1D Plot Group 12.
2
In the Settings window for 1D Plot Group, type Bearing forces in the Label text field.
3
Locate the Title section. From the Title type list, choose Label.
4
Locate the Plot Settings section. Select the y-axis label check box.
5
In the associated text field, type Force (N).
6
Locate the Legend section. From the Position list, choose Lower left.
7
In the Bearing forces toolbar, click  Plot.
8
Click the  Zoom Extents button in the Graphics toolbar.
Animation 1
1
In the Results toolbar, click  Animation and choose Player.
2
In the Settings window for Animation, locate the Scene section.
3
From the Subject list, choose Stress (rotsld).
4
Locate the Frames section. In the Number of frames text field, type 50.