The liquid saturation, sl, which describes the amount of liquid water within the pores, is defined from the specification of a moisture storage function
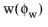
, which specifies the total moisture content (left hand side of the equation below) as a function of the relative humidity
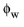
:
where εp (dimensionless) is the porosity.
The default Temperature Τ and
Absolute pressure pA are
User defined. When additional physics interfaces are added to the model, the temperature and absolute pressure variables defined by these physics interfaces can also be selected from the list. For example, if a
Heat Transfer in Building Materials interface is added, you can select
Temperature (ht) from the list. If a
Laminar Flow interface is added, you can select
Absolute pressure (spf) from the list.
If the node was added automatically after selecting the Heat and Moisture Transport predefined multiphysics interface, the temperature of the
Heat and Moisture multiphysics node is used by default and the input field is not editable. To edit the
Temperature field, click
Make All Model Inputs Editable (

).
The Porosity,
εp (a dimensionless number between 0 and 1), uses by default the value
From material. For
User defined the default is 0.
Set the Permeability,
κ (SI unit: m2), to specify the capacity of the porous material to transmit flow. For
User defined select
Isotropic to define a scalar value or
Diagonal,
Symmetric, or
Full to define a tensor value and enter another value or expression in the field or matrix.
Finally, the Moisture storage function 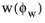
should be set to characterize the relationship between the amount of accumulated water and the relative humidity in the material. The default
Moisture storage function is taken
From material. For
User defined, enter another value or expression.
First, set the Diffusion Coefficient,
D (SI unit: m
2/s), for the binary diffusion of vapor in air in a free medium. The default value 2.6e-5 m²/s is valid at T=298 K and pA=1 atm. You may set a temperature or pressure-dependent diffusion coefficient through the use of the variables
mt.T and
mt.pA.
Then, specify the Effective diffusivity model used to account for the porosity and the tortuosity of the porous medium in the diffusion coefficient,
Deff (SI unit: m
2/s). The available options are
Millington and Quirk model (the default),
Bruggeman model,
Tortuosity model, and
No correction.
For the Tortuosity model option, enter a value for the
Tortuosity factor,
τ (dimensionless). The default is 1.
Finally, set the Velocity field,
ug, that should be interpreted as the Darcy velocity, that is, the volume flow rate per unit cross sectional area. For
User defined enter values or expressions for the components based on space dimensions. Or select an existing velocity field in the component (for example,
Velocity field (br) from a
Brinkman Equations interface).
First, set the Relative liquid water permeability,
κrl (dimensionless), that multiplies the porous medium permeability in the Darcy’s Law to account for the presence of the liquid phase.