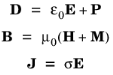To obtain a closed system, the equations include constitutive relations that describe the macroscopic properties of the medium. They are given as:

where ε0 is the permittivity of vacuum, μ0 is the permeability of vacuum, and σ is the electrical conductivity. In the SI units system, the permeability of vacuum is proportional to the dimensionless fine-structure constant and it has an approximate value 4π·10−7 H/m. The velocity of an electromagnetic wave in a vacuum is given as c0 and the permittivity of a vacuum is derived from the relation:

The electromagnetic constants ε0, μ0, and c0 are available in COMSOL Multiphysics as predefined physical constants.

The electric polarization vector P describes how the material is polarized when an electric field E is present. It can be interpreted as the volume density of electric dipole moments. P is generally a function of E. Some materials can have a nonzero P also when there is no electric field present.

The magnetization vector M similarly describes how the material is magnetized when a magnetic field H is present. It can be interpreted as the volume density of magnetic dipole moments. M is generally a function of H. Permanent magnets, for instance, have a nonzero M also when there is no magnetic field present.

For linear materials, the polarization is directly proportional to the electric field, P = ε0 χe E , where χe is the electric susceptibility. Similarly in linear materials, the magnetization is directly proportional to the magnetic field, M = χm H , where χm is the magnetic susceptibility. For such materials, the constitutive relations are:

The parameter εr is the relative permittivity and μr is the relative permeability of the material. Usually these are scalar properties but can, in the general case, be 3-by-3 tensors when the material is anisotropic. The properties ε and μ (without subscripts) are the permittivity and permeability of the material, respectively.

For nonlinear materials, a generalized form of the constitutive relationships is useful. The relationship used for electric fields is D = εoεrE + Dr, where Dr is the remanent displacement, which is the displacement when no electric field is present.

where Br is the remanent magnetic flux density, which is the magnetic flux density when no magnetic field is present.

For some materials, there is a nonlinear relationship between B and H such that

The relation defining the current density is generalized by introducing an externally generated current Je. The resulting constitutive relation is J = σE + Je.