Species Transport Properties

The diffusion coefficients are computed from the following expression based on kinetic gas theory:

(5-13)

Here:

•

Dkj is the binary diffusion coefficient (SI unit: m2/s)

•

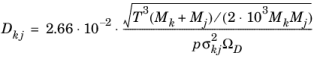
M equals the molecular weight (SI unit: kg/mol)

•

T represents the temperature (SI unit: K)

•

p is the pressure (SI unit: Pa), and

•

σ equals the characteristic length (SI unit: Å) of the Lennard-Jones/Stockmayer potential.

•

In addition, ΩD is the collision integral, given by the following equation (Ref. 2 and Ref. 3):

(5-14)

where

(5-15)

In Equation 5-15, μ is the species dipole moment (SI unit: Debye). For gases at moderate pressure, the binary diffusivity can be used for the multicomponent Maxwell-Stefan diffusivities.

The predefined expression for the dynamic viscosity is given by the kinetic gas theory (Ref. 2 and Ref. 3) as

(5-16)

Here μ represents the dynamic viscosity (SI unit: N·s/m2), and Ωv is the collision integral, given by

with

(5-17)

In Equation 5-17, μ is the species dipole moment (SI unit: Debye). The predefined expression for thermal conductivity comes from the Stiel-Thodos equation (Ref. 4)

(5-18)

where the thermal conductivity k is in (SI unit: W/(m·K)). Here Cp denotes the heat capacity (SI unit: J/(mol·K)).