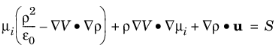Charge Transport Theory
The Charge Transport interface solves for the space charge density of one type of charge carrier (i.e. positive or negative ions) in the presence of electric fields and fluid convection. This interface is design to describe the ion density in the transport region of a corona discharge. The Corona Discharge interface consists of the coupling of the Charge Transport and the Electrostatics interfaces where the electric field and potential at the corona electrode can be set using the Electrode multiphysics coupling feature. The imposition of the electric field and potential at a boundary requires a special formulation of the charge transport equations described below. The charge conservation, current density, and Poisson’s equations are the starting point
(8-1)
(8-2)
(8-3).
This set of equations is manipulated to obtain the equation used to describe the space charge density
(8-4).
where:
•
J is the current density (SI unit: C/m2).
•
ρ is the space charge density (SI unit: C/m3).
•
S is the current source (SI unit: C/m3).
•
Zq is the charge number (SI unit: 1).
•
E is the electric field (SI unit: V/m).
•
V is the electric field (SI unit: V/m).
•
μi is the ion mobility (SI unit: m2/(V.s)).
•
u is the neutral fluid velocity vector (SI unit: m/s). This usually comes from a Navier-Stokes interface.