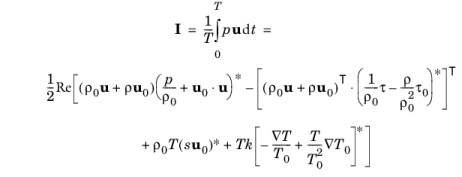
|
phys_id.I_mag
|
|
|
phys_id.Ix
|
|
|
phys_id.Iy
|
|
|
phys_id.Iz
|
|
|
phys_id.Ii_mag
|
|
|
phys_id.Iix
|
|
|
phys_id.Iiy
|
|
|
phys_id.Iiz
|
|
phys_id.I_mag
|
|
|
phys_id.Ir
|
|
|
phys_id.Iz
|
|
|
phys_id.Ii_mag
|
|
|
phys_id.Iir
|
|
|
phys_id.Iiz
|
|
phys_id.I_mag
|
|
|
phys_id.Ix
|
|
|
phys_id.Iy
|
|
|
phys_id.Ii_mag
|
|
|
phys_id.Iix
|
|
|
phys_id.Iiy
|