One possible extension of the plane strain formulation is to assume that the strains are independent of the out-of -plane coordinate z, i.e.

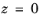
where a, b, and c are constants. Thus, at the cross-section , one has

The above conditions differ from the plane strain state only by the fact that the normal out-of -plane strain component can vary linearly throughout the cross section. This approximation is good when the structure is free to expand in the out-of-plane direction, and the possible bending curvature is small with respect to the extents of the structure in the xy-plane.

At the cross section z = 0, the deformation is in-plane and fully characterized by the in-plane displacement components u(x,y) and v(x,y).

In COMSOL Multiphysics, the coefficients a, b, and c in the expression for the εz strain are modeled as extra degrees of freedom that are constant throughout the model (global variables).

Coefficients a, b, and c are assumed to be small. Then, using the above displacement field in the strain tensor expression and dropping quadratic and higher order terms in the coefficients, one obtains:

Thus, in the leading order approximation, the strains become independent of the out-of-plane coordinate Z.