where 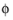(SI unit: m2/s) is the velocity potential of the fluid, a is its amplitude of the velocity potential, and Ψ (SI unit: rad) is the phase. When the distance from any source is many orders of magnitude larger than the wavelength of the acoustic wave, the wave may be assumed to be locally plane; that is,

where k (SI unit: rad/m) is the wave vector, q (SI unit: m) is the position vector, c (SI unit: m/s) is the speed of sound in the medium, and t (SI unit: s) is the time.

While the velocity potential is defined with a stationary fluid in mind, it is possible to apply the same treatment to a homogeneous fluid moving at velocity u (SI unit: m/s) by first formulating the equation of the acoustic wave in a coordinate system in which the fluid is stationary. This results in a more general form of the acoustic wave equation,

This is a mixed time-frequency formulation that has many advantages. See, for example, Ref. 1 and Ref. 5 for further details. It allows a ray acoustic problem to be broken down into its Fourier components, solving for the propagation of one frequency component per ray. Another benefit is the simple use of frequency dependent boundary conditions.