If a 2D plane strain model represents a cross-section of the structure that has a significant uniform extension in the out-of-plane Z-direction, the following 3D solution can be sought in form of the amplitude expansion:

The second part of the solution presents a time-harmonic linear perturbation with an amplitude that can be a function of the in-plane coordinates. Such a perturbation can be seen as an out-of -plane wave, with a small amplitude that propagates in the Z-direction, and has a wave length L and phase velocity c:

There are two alternative approaches. The wave length, and thus the wave number kZ, can be considered as a parameter. Then, ω can be computed by an eigenfrequency analysis for the 2D cross-section with all three displacement components taken as dependent variables. As a result, one obtains

Alternatively, the frequency f (and thus ω) can be taken as a parameter. Then, the solution can be computed via eigenvalue analysis with respect to the wave number kZ using the 2D cross section geometry. Hence,

A standard 2D axially symmetric representation of the structure geometry assumes zero twist displacement component together with independence of the circumferential position φ. The following 3D solution form represent an extension of these assumptions:

where m a circumferential mode number that can only have integer values to obey the axially symmetric nature of the corresponding 3D problem. Thus,

The circumferential wave number can be introduced as .

The static prestress solution has zero twist component

The displacement vector 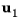can have non-zero values in all three components, which are functions of the radial and axial positions. For a given circumferential mode number m, it can be found using an eigenfrequency analysis in a 2D axially symmetric geometry. Hence,