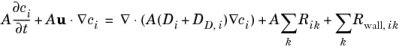The Transport of Diluted Species in Pipes Interface theory is described in this section. This physics interface solves a mass balance equation for Pipes in order to compute the concentration distribution of a solute in a dilute solution, taking the flow velocity as input.

The mass transport equation for a diluted species i an incompressible fluid flowing in a pipe is:

where A (SI unit: m2) is the cross section area available for flow, ci (SI unit: mol/m3) is the diluted species concentration, and u a velocity field. Further, Di (SI unit: m2/s) is the species diffusion coefficient and DD,i (SI unit: m2/s) is the species dispersion coefficient. The second term on the right hand side, Rik (SI unit: mol/(m3·s)), corresponds a source or sink due to chemical reaction number k for species i. Finally, Rwall,ik (SI unit: mol/(m·s)), is a source term due to mass transfer contribution k through the pipe wall.

For laminar flow in circular straight pipes, the total dispersion is given by the sum of molecular diffusion, Di (SI unit: m2/s), and the effect of the velocity profile causing some fractions of an initial plane of fluid in the pipe to move faster than others. COMSOL Multiphysics uses the Taylor (Ref. 21) correlation for this second contribution, DD,i (SI unit: m2/s):

where d is the pipe diameter and L a characteristic pipe length. For turbulent conditions Taylor (Ref. 22) suggests:

For non-Newtonian fluids in the laminar regime Fan (Ref. 23) extended the analysis of Taylor:

with k for Power-law fluids given by:

and k for Bingham plastic fluids:

The parameter φ0 is

where R is the pipe radius and r0 is the radius of the plug flow region in the plastic flow, defined as