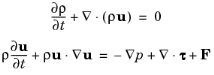See Theory for the Single-Phase Flow Interfaces and Theory for the Turbulent Flow Interfaces in the CFD Module User’s Guide for a description of the theory related to laminar and turbulent single-phase flow interfaces.

It also solves the heat equation, which for a fluid is given in Equation 4-16 by

The work done by pressure changes term

and the viscous heating term

are not included by default because they are usually negligible. These terms can, however, be added by selecting corresponding check boxes in the Nonisothermal Flow feature.

The physics interface also supports heat transfer in solids (Equation 4-14):

where Qted is the thermoelastic damping heat source (SI unit: W/(m3)). This term is not included by default but must be added by selecting the corresponding check box.

Equations for compressible turbulence are derived using the Favre average. The Favre average of a variable T is denoted and is defined by

where H is the enthalpy. The vector

is the laminar, viscous stress tensor. Notice that the thermal conductivity is denoted λ.

is modeled using the Boussinesq approximation:

where k is the turbulent kinetic energy, which in turn is defined by

The correlation between uj″ and H″ in Equation 4-87 is the turbulent transport of heat. It is modeled analogously to the laminar conductive heat flux

are modeled by a generalization of the molecular diffusion and turbulent transport terms found in the incompressible k equation

Inserting Equation 4-88, Equation 4-89, Equation 4-90, Equation 4-91 and Equation 4-92 into Equation 4-87 gives

The Favre average can also be applied to the momentum equation, which, using Equation 4-89, can be written

Taking the inner product between 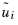and Equation 4-94 results in an equation for the resolved kinetic energy, which can be subtracted from Equation 4-93 with the following result:

According to Wilcox (Ref. 24), it is usually a good approximation to neglect the contributions of k for flows with Mach numbers up to the supersonic range. This gives the following approximation of Equation 4-95:

Larsson (Ref. 25) suggests to make the split

Equation 4-97 is completely analogous to the laminar energy equation of Equation 4-13 and can be expanded using the same theory to get the temperature equation similar to Equation 4-16 (see for example Ref. 25):

This is a relatively exact model for PrT, while still quite simple. In Ref. 26, it is compared to other models for PrT and found to be a good approximation for most kinds of turbulent wall bounded flows except for turbulent flow of liquid metals. The model is given by

where the Prandtl number at infinity is PrT∞ = 0.85 and λ is the conductivity.

Weigand et al. (Ref. 27) suggested an extension of Equation 4-98 to liquid metals by introducing

where Re∞, the Reynolds number at infinity must be provided either as a constant or as a function of the flow field. This is entered in the Model Inputs section of the Fluid feature.

Analogous to the single-phase flow wall functions (see Wall Functions described for the Wall boundary condition), there is a theoretical gap between the solid wall and the computational domain for the fluid and temperature fields. This gap is often ignored when the computational geometry is drawn.

The heat flux between the fluid with temperature Tf and a wall with temperature Tw, is:

where ρ is the fluid density, Cp is the fluid heat capacity, Cμ is a turbulence modeling constant, and k is the turbulent kinetic energy. T+ is the dimensionless temperature and is given by (Ref. 28):

λ is the thermal conductivity, and κ is the von Karman constant equal to 0.41.

The computational results should be checked so that the distance between the computational fluid domain and the wall, δw, is everywhere small compared to any geometrical quantity of interest. The distance δw is available for evaluation on boundaries.

where H0 is the total enthalpy.