Theory for Specific Current Distribution Feature Nodes
Electrolyte Theory
The Electrolyte node defines a current balance in the electrolyte. The domain equation is:
where il denotes the current density vector. In free electrolyte, there is no source or sink of charge.
The definition of the current density vector depends on the equation formulation of the electrolyte charge transport, as discussed above in Domain Equations for Primary and Secondary Current Distributions and Domain Equations for Tertiary Current Distributions Using the Nernst-Planck Equations and Electroneutrality.
•
For the Primary and Secondary Current Distribution interfaces, see Electrolyte.
•
For the Tertiary Current Distribution, Nernst-Planck interface, see Electrolyte.
Porous Electrode Theory
A porous electrode is a mixed material with one electrode phase and one electrolyte phase. (A special case of a porous electrode is the gas diffusion electrode, as found in a fuel cell. These contain a gas pore phase which is inert to charge transfer.) To model a porous electrode we define two separate current balances according to the following equations:
and
In these equations, il denotes the current density vector in the electrolyte, as discussed above in Domain Equations for Primary and Secondary Current Distributions and Domain Equations for Tertiary Current Distributions Using the Nernst-Planck Equations and Electroneutrality.
In addition to the current balances, it is necessary to also formulate mass balance equations for the species in the electrolyte phase for the tertiary case.
It is also common to used corrected conductivities and diffusion parameter values in a porous electrode to account for the lowered volume fraction of the conducting phase, and the longer transport distance due to the tortuosity of the pores.
The current balances in the pore electrolyte and in the electrode matrix contain sources and sinks according to the charge transfer reactions that take place in the electrode catalyst. For example, if only one charge transfer reaction takes place in the porous electrode and the rate of this reaction is described by the Butler-Volmer equation, the domain equations are the following:
where Av denotes the specific surface area (dimension L2/L3), and η the overpotential according to
and Eeq denotes the equilibrium potential for the charge transfer reaction.
If the porous electrode is a cathode, then the charge transfer reaction is a source for the current balance in the electrode, because it receives current from the pore electrolyte. The charge transfer reaction is then a sink for the current balance in the pore electrolyte, because the current is transferred from the pore electrolyte to the electrode in a cathodic reaction.
The corresponding sources and sinks in the current balances that are due to the charge transfer reactions are also coupled to the material balances for the charged species. This means that the exemplar Butler-Volmer expression above is also included in the material balances as a reaction term, Ri, by using Faraday’s laws for each of the species that take part in charge transfer reactions.
•
For the Primary and Secondary Current Distribution interfaces, see Porous Electrode.
•
For the Tertiary Current Distribution, Nernst-Planck interface, see Porous Electrode.
Electrode Reactions Theory
Charge transfer reactions occurring at an interface between an electrode and an electrolyte domain gives rise to a normal current flux that equals the sum of all reaction currents according to
where iloc,m (A/m2) is the Electrode Reaction current density of the charge transfer electrode reaction of index m, il the current density vector in the electrolyte and is the current density vector in the electrode.
Porous Electrode Reactions Theory
For a porous electrode, the electrode reaction current densities are multiplied by the surface area to yield a source or sink in the current balance domain equation according to:
where Av is the specific surface area of the electrocatalyst.
Porous Electrode Reaction
Electrode Theory
Electron conduction in an Electrode is modeled using Ohm’s law. The domain equation is the following:
where is denotes the current density vector according to:
and where σs denotes the electrical conductivity and 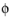s the electrolyte potential.
Electrolyte Current Density Theory
An applied current density can be defined as its component perpendicular to the boundary according to:
The current density can also be defined including all its components:
where il, bnd is a given expression for the current density vector.
Electrolyte Current Density
Electrolyte Current Theory
The Electrode Current boundary condition sets the total current at a given position in the electrolyte without imposing a current density distribution. The conditions yields a constant electrolyte potential, along the given boundary, that satisfies the total value of the current. The boundary condition is a good choice in the middle of a cell with planar electrodes, where the isopotential level can be a plane (or close to a plane in 3D, or line in 2D) but where the current density distribution is unknown.
The feature adds one unknown variable, the electrolyte potential, 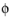l, bnd, along the boundary. It then adds one additional equation for the total current, which is an integral over the boundary:
The average current density condition imposes the same equation but multiplies the current density by the area of the boundary to obtain the value of the total current In, l.
Electrode Current Theory
The Electrode Current adds one unknown variable, the electric potential, 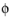s, bnd, along the boundary. It then adds one additional equation for the total current, which is an integral over the boundary:
where
and σs denotes the electrode conductivity and 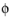s the electric potential. The average current density condition imposes the same equation but multiplies the current density by the area of the boundary to obtain the value of the total current, In,s.
Symmetry Theory
The Symmetry boundary condition, in the Primary Current Distribution and Secondary Current Distribution interfaces is identical to the Insulation condition and is expressed according to the equation below.
where ik denotes the current density vector and k = l, s is an index for the electrolyte and electrode, respectively.
The Symmetry boundary condition for the Tertiary Current Distribution, Nernst-Planck interface imposes a no-flux condition for the molar flux of species at a boundary. The condition is expressed as follows:
Electrode Current Density Theory
An applied Electrode Current Density can be defined as its component perpendicular to the boundary according to:
where
and σs denotes the electrode conductivity and 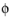s the electric potential.
The current density can also be defined including all its components:
where is, bnd is a given expression for the current density vector.
Electrode Power Theory
The Electrode Power boundary condition is used to specify either the total electrode power or the average electrode power density drawn from or inserted into an electrochemical cell at an electrode boundary.
For a total power condition, the boundary electric potential of an electrode is set to a potential s, bnd, defined by the condition for the total power on the boundary according to:
where 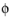s, ground is the ground potential of the cell, and Ptotal (W) is the power to be drawn.
For an average power condition, Ptotal is calculated by:
where Pavg is the average power density on the boundary, and A is the boundary area.
For a galvanic cell, such as a battery during discharge or a fuel cell, there is a maximum power level, beyond which a further current increase causes a lowered output power due to increasing voltage losses. A result of this is that there can be two existing solutions for the same power setting. In these cases the choice of initial values determines the final solution.
Electrode Power
Ion Exchange Membrane Boundary Theory
In an ion exchange membrane the concentration of charge carriers is fixed, whereas charge carrying species are free to move in a liquid electrolyte. To preserve continuity of the electrochemical potential over a boundary between an ion exchange membrane and a free electrolyte, a potential shift of the electrolyte potential arises if the ion concentrations in the two domains are different.
At the boundary between a liquid electrolyte phase and a ion-exchange membrane phase, denoted l and m, the Donnan potential, Δφ(V), describes the relation between the concentration of a species, cl and cm (mol/m3), at each side of the boundary and the electrolyte potentials φl and φm:
where T(K) is the temperature, R (mol/(J K)) the molar gas constant, z the species charge and F(C/mol) is Faraday's constant.
The current densities Il and Im in the normal direction n on each side of the boundary are equal so that the current density over the membrane-liquid interface is continuous:
The molar flux Nl of the charge carrying species in the liquid electrolyte is set proportional to the current density according to Faraday's law:
For all other species the molar flux is zero.
Thin Insulating Layer Theory
The usual approach to adding an insulating body in a model is to add a domain to the geometry and then exclude this domain from the electrolyte. An insulating boundary condition on the boundaries of the insulating body is then used according to
If the model also includes mass transport, no flux conditions are also used for each species on the insulation boundaries.
Introducing very thin domains in the model geometry may however cause issues with meshing and significantly increase the memory requirements during solving, since the thin thicknesses of the layers need to be resolved in the mesh.
As an alternative to describing the thin layers as domains in the geometry, the Thin Insulating Layer feature instead models the thin layer as a boundary, i.e. a layer of infinitely small thickness, and then mathematically "slits" the dependent variables. The slitting implies that separate degrees of freedoms are used on each side on the boundary. The same boundary conditions as above are then used on each side of the boundary.
Thin Electrode Layer