Using a Fick’s law approximation, the relative mass flux due to molecular diffusion is assumed to be governed by

where 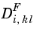represents a general diffusion matrix (SI unit: m2/s) describing the diffusion of species i into the mixture. This form makes it possible to use any diffusion coefficient, matrix, or empirical model based on Fick’s law. For example, in situations when the mass transport is not dominated by diffusion, an alternative is to use the diffusion coefficients at infinite dilution,

When using multicomponent diffusivities based on Fick’s law, as described above, or when using mixture-averaged coefficients, the sum of the relative mass fluxes is not strictly constrained to zero. To reduce the relative error it is preferable to choose the species with the highest mass fraction as the species that is not solved for, and which is instead computed from the mass conservation constraint in Equation 8-4. It is not always necessary to know in advance which species has the highest mass fraction—it is possible to change the species solved for by the mass conservation constraint in Equation 8-4.