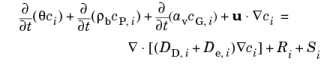The following equations for the concentrations, ci, describe the transport of solutes in a variably saturated porous medium for the most general case, when the pore space is primarily filled with liquid but also contain pockets or immobile gas:

On the left-hand side of Equation 5-17, the first three terms correspond to the accumulation of species within the liquid, solid, and gas phases, while the last term describes the convection due to the velocity field u (SI unit: m/s).

In Equation 5-17 ci denotes the concentration of species i in the liquid (SI unit: mol/m3), cP, i the amount adsorbed to (or desorbed from) solid particles (moles per unit dry weight of the solid), and cG, i the concentration of species i in the gas phase.

The equation balances the mass transport throughout the porous medium using the porosity εp, the liquid volume fraction θ; the bulk (or drained) density, ρb = (1 − εp)ρ, and the solid phase density ρ (SI unit: kg/m3).

For saturated porous media, the liquid volume fraction θ is equal to the porosity εp, but for partially saturated porous media, they are related by the saturation s as θ = sεp. The resulting gas volume fraction is av = εp − θ = (1-s)εp.

On the right-hand side of Equation 5-17, the first term introduces the spreading of species due to mechanical mixing as well as from diffusion and volatilization to the gas phase. The tensor is denoted DD (SI unit: m2/s) and the effective diffusion by De (SI unit: m2/s).

The last two terms on the right-hand side of Equation 5-17 describe production or consumption of the species; Ri is a reaction rate expression which can account for reactions in the liquid, solid, or gas phase, and Si is an arbitrary source term, for example due to a fluid flow source or sink.

In order to solve for the solute concentration of species i, ci, the solute mass sorbed to solids cP,i and dissolved in the gas-phase cG,i are assumed to be functions of ci. Expanding the time-dependent terms gives

where kP,i = ∂cP,i/∂ci is the adsorption isotherm and kG,i = ∂cG,i/∂ci is the linear volatilization. Equation 5-17 can then be written as

In the case of transport in a saturated porous medium, θ = εp and the governing equations are